| << Chapter < Page | Chapter >> Page > |

The first principle to understand about parallel circuits is that the voltage is equal across all components in the circuit. This is because there are only two sets of electrically common points in a parallel circuit, and voltage measured between sets of common points must always be the same at any given time. So, for the circuit shown, the following is true:
The second principle for a parallel circuit is that all the currents through each resistor must add up to the total current in the circuit.
Also, from applying Ohm's Law to the entire circuit, we can write:
where is the equivalent resistance in this parallel arrangement.
We are now ready to apply Ohm's Law to each resistor, to get:
This can be also written as:
Now we have:
For resistors in parallel, the equivalent resistance is:
Let us apply this formula to the following circuit.
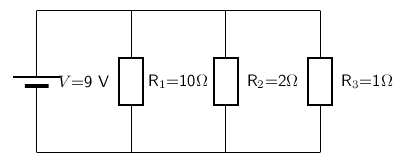
What is the total resistance in the circuit?
Khan academy video on electric circuits 2
Khan academy video on electric circuits 3
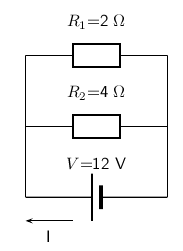
Calculate the current ( ) in this circuit if the resistors are both ohmic in nature.
We are required to calculate the current flowing in the circuit.
Since the resistors are Ohmic in nature, we can use Ohm's Law. There are however two resistors in the circuit and we need to find the total resistance.
Since the resistors are connected in series, the total resistance is:
Therefore,
A 2 A current is flowing in the circuit.
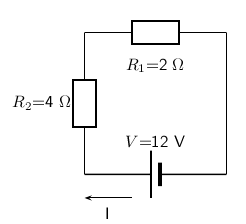
Calculate the current ( ) in this circuit if the resistors are both ohmic in nature.
We are required to calculate the current flowing in the circuit.
Since the resistors are Ohmic in nature, we can use Ohm's Law. There are however two resistors in the circuit and we need to find the total resistance.
Since the resistors are connected in parallel, the total resistance is:
Therefore,
A 9 A current is flowing in the circuit.
Two ohmic resistors ( and ) are connected in series with a cell. Find the resistance of , given that the current flowing through and is 0,25 A and that the voltage across the cell is 1,5 V. =1 .
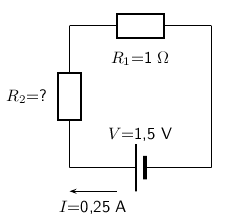
We can use Ohm's Law to find the total resistance in the circuit, and then calculate the unknown resistance using:
because it is in a series circuit.
We know that:
and that
Since
Therefore,
Real batteries are made from materials which have resistance. This means that real batteries are not just sources of potential difference (voltage), but they also possess internal resistance. If the total voltage source is referred to as the emf, , then a real battery can be represented as an emf connected in series with a resistor . The internal resistance of the battery is represented by the symbol .
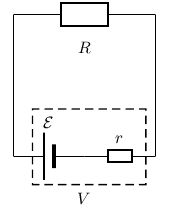
The external resistance in the circuit is referred to as the load.
Suppose that the battery with emf and internal resistance supplies a current through an external load resistor . Then the voltage drop across the load resistor is that supplied by the battery:
Similarly, from Ohm's Law, the voltage drop across the internal resistance is:
The voltage of the battery is related to its emf and internal resistance by:
The emf of a battery is essentially constant because it only depends on the chemical reaction (that converts chemical energy into electrical energy) going on inside the battery. Therefore, we can see that the voltage across the terminals of the battery is dependent on the current drawn by the load. The higher the current, the lower the voltage across the terminals, because the emf is constant. By the same reasoning, the voltage only equals the emf when the current is very small.
The maximum current that can be drawn from a battery is limited by a critical value . At a current of , =0 V. Then, the equation becomes:
The maximum current that can be drawn from a battery is less than .
What is the internal resistance of a battery if its emf is 12 V and the voltage drop across its terminals is 10 V when a current of 4 A flows in the circuit when it is connected across a load?
It is an internal resistance problem. So we use the equation:
The internal resistance of the resistor is 0.5 .


Notification Switch
Would you like to follow the 'Siyavula textbooks: grade 11 physical science' conversation and receive update notifications?