1. Home
  2. University physics volume 1
  3. Unit 2. waves and acoustics
  4. Oscillations
  5. Simple harmonic motion

15.1 Simple harmonic motion  (Page 6/16)

<< Chapter < Page
  University physics volume 1     Page 6 / 16
Chapter >> Page >
F net = k y − k y 0 − ( k y 0 − k y 1 ) = − k ( y − y 1 ) .

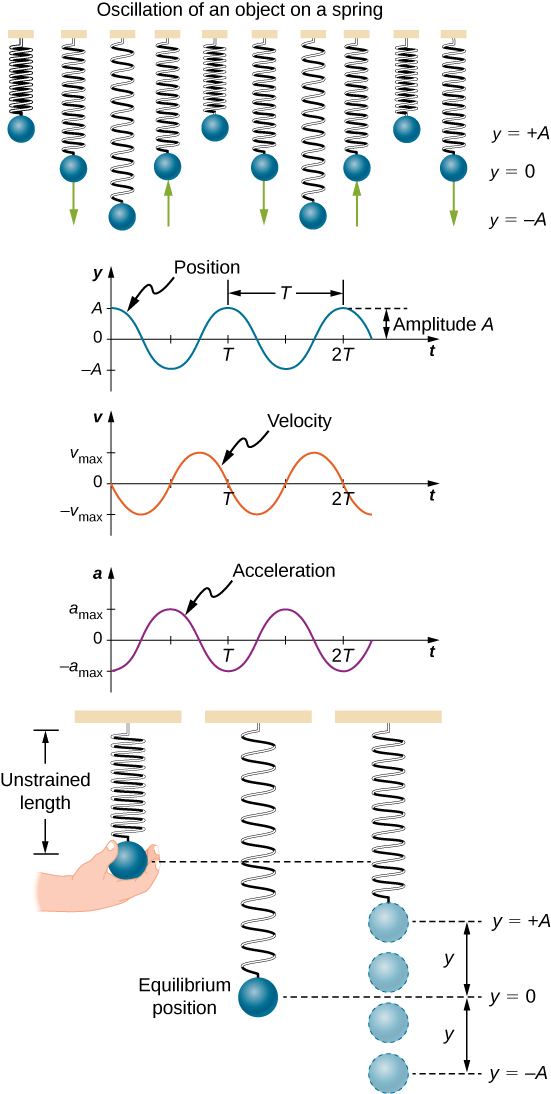
Recall that y 1 is just the equilibrium position and any position can be set to be the point y = 0.00 m . So let’s set y 1 to y = 0.00 m . The net force then becomes

F net = − k y ; m d 2 y d t 2 = − k y .

This is just what we found previously for a horizontally sliding mass on a spring. The constant force of gravity only served to shift the equilibrium location of the mass. Therefore, the solution should be the same form as for a block on a horizontal spring, y ( t ) = A cos ( ω t + ϕ ) . The equations for the velocity and the acceleration also have the same form as for the horizontal case. Note that the inclusion of the phase shift means that the motion can actually be modeled using either a cosine or a sine function, since these two functions only differ by a phase shift.

A series of 10 illustrations of a ball, attached to a vertical spring, is shown. The illustrations are displayed next to each other, with the tops of the springs aligned. The vertical positions y = + A, y = 0, and y = -A are labeled on the right. Working our way from left to right: In the left-most drawing, the spring is compressed so the ball is at y = + A and at rest. In the second drawing, the ball is at y = 0 and is moving downward. In the third drawing, the spring is stretched so that the ball is at y = - A and at rest. In the fourth drawing, the ball is at y = 0 and is moving upward. In the fifth drawing, the spring is compressed so the ball is at y = + A and at rest. In the sixth drawing, the ball is at y = 0 and is moving downward. In the seventh drawing, the spring is stretched so that the ball is at y = - A and at rest. In the eighth drawing, the ball is at y = 0 and is moving upward. In the ninth drawing, the spring is compressed so the ball is at y = + A and at rest. In the tenth drawing, the ball is at y = 0 and is moving downward. Below these illustrations is a series of graphs, aligned vertically. The top graph is of position as a function of time. The vertical axis is position y, with a range of – A to +A. The horizontal axis is time t, labeled in increments of T. The graph has value y=+A at t=0 and oscillates two and one quarter cycles. The horizontal distance between maxima is labeled as T and the vertical distance between the horizontal axis and the maximum is labeled as amplitude A. The middle graph is of velocity as a function of time. The vertical axis is velocity v, with a range of minus v sub max to v max. The horizontal axis is time t, labeled in increments of T. The graph has value v=0 and a negative slope at t=0, and oscillates two and one quarter cycles. The bottom graph is of acceleration as a function of time. The vertical axis is acceleration a, with a range of minus a sub max to a max. The horizontal axis is time t, labeled in increments of T. The graph has value a equals minus a sub max and a and oscillates two and one quarter cycles. Below the graphs are three illustrations of the ball on the spring. The positions y = + A, y=0, and y = -A are labeled on the right. In the leftmost diagram, a hand holds the ball and the length of the spring is labeled as the unstrained length. This position is above the y = + A position. In the middle picture, the ball is not being held and is at a lower position labeled as the equilibrium position. This position is y = 0. In the rightmost diagram, the ball is shown in four different positions. These positions are y = + A, just above y = 0, just below y = 0 , and at y = -A. The spring is shown only with its bottom attached to the ball at the y = + A position.
Graphs of y ( t ), v ( t ), and a ( t ) versus t for the motion of an object on a vertical spring. The net force on the object can be described by Hooke’s law, so the object undergoes SHM. Note that the initial position has the vertical displacement at its maximum value A ; v is initially zero and then negative as the object moves down; the initial acceleration is negative, back toward the equilibrium position and becomes zero at that point.

Summary

  • Periodic motion is a repeating oscillation. The time for one oscillation is the period T and the number of oscillations per unit time is the frequency f . These quantities are related by f = 1 T .
  • Simple harmonic motion (SHM) is oscillatory motion for a system where the restoring force is proportional to the displacement and acts in the direction opposite to the displacement.
  • Maximum displacement is the amplitude A . The angular frequency ω , period T , and frequency f of a simple harmonic oscillator are given by ω = k m , T = 2 π m k , and f = 1 2 π k m , where m is the mass of the system and k is the force constant.
  • Displacement as a function of time in SHM is given by x ( t ) = A cos ( 2 π T t + ϕ ) = A cos ( ω t + ϕ ) .
  • The velocity is given by v ( t ) = − A ω sin ( ω t + ϕ ) = − v max sin ( ω t + ϕ ) , where v max = A ω = A k m .
  • The acceleration is a ( t ) = − A ω 2 cos ( ω t + ϕ ) = − a max cos ( ω t + ϕ ) , where a max = A ω 2 = A k m .

Conceptual questions

What conditions must be met to produce SHM?

The restoring force must be proportional to the displacement and act opposite to the direction of motion with no drag forces or friction. The frequency of oscillation does not depend on the amplitude.

Got questions? Get instant answers now!

(a) If frequency is not constant for some oscillation, can the oscillation be SHM? (b) Can you think of any examples of harmonic motion where the frequency may depend on the amplitude?

Got questions? Get instant answers now!

Give an example of a simple harmonic oscillator, specifically noting how its frequency is independent of amplitude.

Examples: Mass attached to a spring on a frictionless table, a mass hanging from a string, a simple pendulum with a small amplitude of motion. All of these examples have frequencies of oscillation that are independent of amplitude.

Got questions? Get instant answers now!

Explain why you expect an object made of a stiff material to vibrate at a higher frequency than a similar object made of a more pliable material.

Got questions? Get instant answers now!

As you pass a freight truck with a trailer on a highway, you notice that its trailer is bouncing up and down slowly. Is it more likely that the trailer is heavily loaded or nearly empty? Explain your answer.

Since the frequency is proportional to the square root of the force constant and inversely proportional to the square root of the mass, it is likely that the truck is heavily loaded, since the force constant would be the same whether the truck is empty or heavily loaded.

Got questions? Get instant answers now!

Some people modify cars to be much closer to the ground than when manufactured. Should they install stiffer springs? Explain your answer.

Got questions? Get instant answers now!

Problems

Prove that using x ( t ) = A sin ( ω t + ϕ ) will produce the same results for the period for the oscillations of a mass and a spring. Why do you think the cosine function was chosen?

Proof

Got questions? Get instant answers now!

What is the period of 60.0 Hz of electrical power?

Got questions? Get instant answers now!

If your heart rate is 150 beats per minute during strenuous exercise, what is the time per beat in units of seconds?

0.400 s/beat

Got questions? Get instant answers now!

Find the frequency of a tuning fork that takes 2.50 × 10 −3 s to complete one oscillation.

Got questions? Get instant answers now!

A stroboscope is set to flash every 8.00 × 10 −5 s . What is the frequency of the flashes?

12,500 Hz

Got questions? Get instant answers now!

A tire has a tread pattern with a crevice every 2.00 cm. Each crevice makes a single vibration as the tire moves. What is the frequency of these vibrations if the car moves at 30.0 m/s?

Got questions? Get instant answers now!

Each piston of an engine makes a sharp sound every other revolution of the engine. (a) How fast is a race car going if its eight-cylinder engine emits a sound of frequency 750 Hz, given that the engine makes 2000 revolutions per kilometer? (b) At how many revolutions per minute is the engine rotating?

a. 340 km/hr; b. 11.3 × 10 3 rev/min

Got questions? Get instant answers now!

A type of cuckoo clock keeps time by having a mass bouncing on a spring, usually something cute like a cherub in a chair. What force constant is needed to produce a period of 0.500 s for a 0.0150-kg mass?

Got questions? Get instant answers now!

A mass m 0 is attached to a spring and hung vertically. The mass is raised a short distance in the vertical direction and released. The mass oscillates with a frequency f 0 . If the mass is replaced with a mass nine times as large, and the experiment was repeated, what would be the frequency of the oscillations in terms of f 0 ?

f = 1 3 f 0

Got questions? Get instant answers now!

A 0.500-kg mass suspended from a spring oscillates with a period of 1.50 s. How much mass must be added to the object to change the period to 2.00 s?

Got questions? Get instant answers now!

By how much leeway (both percentage and mass) would you have in the selection of the mass of the object in the previous problem if you did not wish the new period to be greater than 2.01 s or less than 1.99 s?

0.009 kg; 2%

Got questions? Get instant answers now!

Questions & Answers

A golfer on a fairway is 70 m away from the green, which sits below the level of the fairway by 20 m. If the golfer hits the ball at an angle of 40° with an initial speed of 20 m/s, how close to the green does she come?
Aislinn Reply
cm
tijani
what is titration
John Reply
what is physics
Siyaka Reply
A mouse of mass 200 g falls 100 m down a vertical mine shaft and lands at the bottom with a speed of 8.0 m/s. During its fall, how much work is done on the mouse by air resistance
Jude Reply
Can you compute that for me. Ty
Jude
what is the dimension formula of energy?
David Reply
what is viscosity?
David
what is inorganic
emma Reply
what is chemistry
Youesf Reply
what is inorganic
emma
Chemistry is a branch of science that deals with the study of matter,it composition,it structure and the changes it undergoes
Adjei
please, I'm a physics student and I need help in physics
Adjanou
chemistry could also be understood like the sexual attraction/repulsion of the male and female elements. the reaction varies depending on the energy differences of each given gender. + masculine -female.
Pedro
A ball is thrown straight up.it passes a 2.0m high window 7.50 m off the ground on it path up and takes 1.30 s to go past the window.what was the ball initial velocity
Krampah Reply
2. A sled plus passenger with total mass 50 kg is pulled 20 m across the snow (0.20) at constant velocity by a force directed 25° above the horizontal. Calculate (a) the work of the applied force, (b) the work of friction, and (c) the total work.
Sahid Reply
you have been hired as an espert witness in a court case involving an automobile accident. the accident involved car A of mass 1500kg which crashed into stationary car B of mass 1100kg. the driver of car A applied his brakes 15 m before he skidded and crashed into car B. after the collision, car A s
Samuel Reply
can someone explain to me, an ignorant high school student, why the trend of the graph doesn't follow the fact that the higher frequency a sound wave is, the more power it is, hence, making me think the phons output would follow this general trend?
Joseph Reply
Nevermind i just realied that the graph is the phons output for a person with normal hearing and not just the phons output of the sound waves power, I should read the entire thing next time
Joseph
Follow up question, does anyone know where I can find a graph that accuretly depicts the actual relative "power" output of sound over its frequency instead of just humans hearing
Joseph
"Generation of electrical energy from sound energy | IEEE Conference Publication | IEEE Xplore" ***ieeexplore.ieee.org/document/7150687?reload=true
Ryan
what's motion
Maurice Reply
what are the types of wave
Maurice
answer
Magreth
progressive wave
Magreth
hello friend how are you
Muhammad Reply
fine, how about you?
Mohammed
hi
Mujahid
A string is 3.00 m long with a mass of 5.00 g. The string is held taut with a tension of 500.00 N applied to the string. A pulse is sent down the string. How long does it take the pulse to travel the 3.00 m of the string?
yasuo Reply
Who can show me the full solution in this problem?
Reofrir Reply
<< Chapter < Page Page > Chapter >>
Practice Key Terms 10

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

Get it on Google Play Download on the App Store Now




Source:  OpenStax, University physics volume 1. OpenStax CNX. Sep 19, 2016 Download for free at http://cnx.org/content/col12031/1.5
Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'University physics volume 1' conversation and receive update notifications?

Ask