9.3 Conservation of linear momentum (Page 11/10)

University physics volume 1 Page 1 / 10

By the end of this section, you will be able to:

Explain the meaning of “conservation of momentum”
Correctly identify if a system is, or is not, closed
Define a system whose momentum is conserved
Mathematically express conservation of momentum for a given system
Calculate an unknown quantity using conservation of momentum

Recall Newton’s third law: When two objects of masses $m_{1}$ and $m_{2}$ interact (meaning that they apply forces on each other), the force that object 2 applies to object 1 is equal in magnitude and opposite in direction to the force that object 1 applies on object 2. Let:

${\vec{F}}_{21} =$ the force on $m_{1}$ from $m_{2}$
${\vec{F}}_{12} =$ the force on $m_{2}$ from $m_{1}$

Then, in symbols, Newton’s third law says

\begin{matrix} {\vec{F}}_{21} & = & − {\vec{F}}_{12} \\ m_{1} {\vec{a}}_{1} & = & − m_{2} {\vec{a}}_{2} . \end{matrix}

(Recall that these two forces do not cancel because they are applied to different objects. $F_{21}$ causes $m_{1}$ to accelerate, and $F_{12}$ causes $m_{2}$ to accelerate.)

Although the magnitudes of the forces on the objects are the same, the accelerations are not, simply because the masses (in general) are different. Therefore, the changes in velocity of each object are different:

\frac{d {\vec{v}}_{1}}{d t} \neq \frac{d {\vec{v}}_{2}}{d t} .

However, the products of the mass and the change of velocity are equal (in magnitude):

m_{1} \frac{d {\vec{v}}_{1}}{d t} = − m_{2} \frac{d {\vec{v}}_{2}}{d t} .

It’s a good idea, at this point, to make sure you’re clear on the physical meaning of the derivatives in [link] . Because of the interaction, each object ends up getting its velocity changed, by an amount dv . Furthermore, the interaction occurs over a time interval dt , which means that the change of velocities also occurs over dt . This time interval is the same for each object.

Let‘s assume, for the moment, that the masses of the objects do not change during the interaction. (We’ll relax this restriction later.) In that case, we can pull the masses inside the derivatives:

\frac{d}{d t} (m_{1} {\vec{v}}_{1}) = - \frac{d}{d t} (m_{2} {\vec{v}}_{2})

and thus

\frac{d {\vec{p}}_{1}}{d t} = - \frac{d {\vec{p}}_{2}}{d t} .

This says that the rate at which momentum changes is the same for both objects. The masses are different, and the changes of velocity are different, but the rate of change of the product of m and $\vec{v}$ are the same.

Physically, this means that during the interaction of the two objects ( $m_{1} and m_{2}$ ), both objects have their momentum changed; but those changes are identical in magnitude, though opposite in sign. For example, the momentum of object 1 might increase, which means that the momentum of object 2 decreases by exactly the same amount.

In light of this, let’s re-write [link] in a more suggestive form:

\frac{d {\vec{p}}_{1}}{d t} + \frac{d {\vec{p}}_{2}}{d t} = 0 .

This says that during the interaction, although object 1’s momentum changes, and object 2’s momentum also changes, these two changes cancel each other out, so that the total change of momentum of the two objects together is zero.

Since the total combined momentum of the two objects together never changes, then we could write

\frac{d}{d t} ({\vec{p}}_{1} + {\vec{p}}_{2}) = 0

from which it follows that

{\vec{p}}_{1} + {\vec{p}}_{2} = constant .

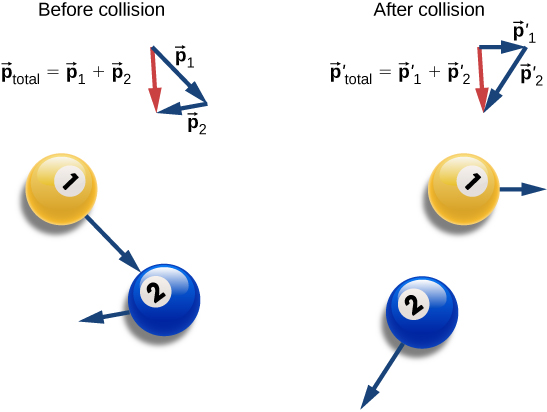
As shown in [link] , the total momentum of the system before and after the collision remains the same.

Before collision yellow ball1 is moving down and to the right, aiming at the center of blue ball 2. Blue ball 2 is moving to the left and slightly down, and more slowly than ball 1. We are told that p total vector equals p 1 vector plus p 2 vector and we are shown the sum as a vector diagram: p 1 and p 2 are placed with the tail of p 2 at the head of p 1. A vector is drawn from the tail of p 1 to the head of p 2. After the collision, the yellow ball is moving slowly to the right and p 2 is moving more rapidly down and to the left. We are told that p prime total vector equals p prime 1 vector plus p prime 2 vector and we are shown the sum as a vector diagram: p prime 1 and p prime 2 are placed with the tail of p prime 2 at the head of p prime 1. A vector is drawn from the tail of p prime 1 to the head of p prime 2 and is the same length and in the same direction as the sum vector before collision. — Before the collision, the two billiard balls travel with momenta ${\vec{p}}_{1}$ and ${\vec{p}}_{3}$ . The total momentum of the system is the sum of these, as shown by the red vector labeled ${\vec{p}}_{total}$ on the left. After the collision, the two billiard balls travel with different momenta ${\vec{p}}^{'}_{1}$ and ${\vec{p}}^{'}_{3}$ . The total momentum, however, has not changed, as shown by the red vector arrow ${\vec{p}}^{'}_{total}$ on the right.

<< Chapter < Page Page > Chapter >>

Practice Key Terms 3

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, University physics volume 1. OpenStax CNX. Sep 19, 2016 Download for free at http://cnx.org/content/col12031/1.5

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'University physics volume 1' conversation and receive update notifications?

Ask

	4 Neuroanatomy 04 Spinal Cord Brain Stem By Stephen Voron Start Quiz
	9 Lec:9 Descriptive Statistics By Janet Forrester Start Quiz
	6 Microbiology Midterm Practice By Madison Christian Start Test
	1 Gastrointestinal Pathophysiology By Laurence Bailen Start Exam
	15 AP 15 Autonomic Nervous System Essay By OpenStax Start Flashcards
©flickr: Gareth	Resume Writing MCQ By Abby Sharp Start Quiz
	Nutrition and Chronic Disease- Test 2 By Madison Christian Start Flashcards
	1 BOD - DERMATOLOGY - By Brooke Delaney Start Exam
	Job Search Skills MCQ By Mary Matera Start Quiz
	4 CDL Quiz - General Knowledge Part 2 By Jazzycazz Jackson Start Quiz