14.1 Fluids, density, and pressure  (Page 5/13)

What force must a dam withstand?

Consider the pressure and force acting on the dam retaining a reservoir of water ( [link] ). Suppose the dam is 500-m wide and the water is 80.0-m deep at the dam, as illustrated below. (a) What is the average pressure on the dam due to the water? (b) Calculate the force exerted against the dam.

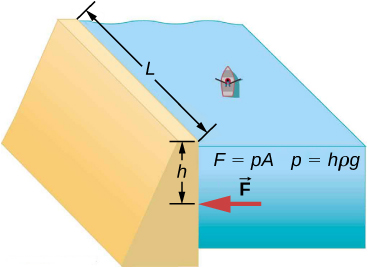
The average pressure p due to the weight of the water is the pressure at the average depth h of 40.0 m, since pressure increases linearly with depth. The force exerted on the dam by the water is the average pressure times the area of contact, $F=pA.$

Solution

1. The average pressure due to the weight of a fluid is
   
   $p=h\rho g.$
   
   Entering the density of water from [link] and taking h to be the average depth of 40.0 m, we obtain
   
   $\begin{array}{cc}\hfill p& =(40.0\text{m})\left({10}^3\frac{\text{kg}}{{\text{m}}^3}\right)\left(9.80\frac{\text{m}}{{\text{s}}^2}\right)\hfill \\ & =3.92\times {10}^5\frac{\text{N}}{{\text{m}}^2}=392\text{kPa}\text{.}\hfill \end{array}$
2. We have already found the value for p . The area of the dam is
   
   $A=80.0\text{m}\times 500\text{m}=4.00\times {10}^4{\text{m}}^2,$
   
   so that
   
   $\begin{array}{}\\ \hfill F& =(3.92\times {10}^5{\text{N/m}}^2)(4.00\times {10}^4{\text{m}}^2)\hfill \\ & =1.57\times {10}^{10}\text{N}\text{.}\hfill \end{array}$

Significance

Although this force seems large, it is small compared with the $1.96\times {10}^{13}\text{N}$ weight of the water in the reservoir. In fact, it is only 0.0800% of the weight.

Got questions? Get instant answers now!

Pressure in a static fluid in a uniform gravitational field

A static fluid is a fluid that is not in motion. At any point within a static fluid, the pressure on all sides must be equal—otherwise, the fluid at that point would react to a net force and accelerate.

The pressure at any point in a static fluid depends only on the depth at that point. As discussed, pressure in a fluid near Earth varies with depth due to the weight of fluid above a particular level. In the above examples, we assumed density to be constant and the average density of the fluid to be a good representation of the density. This is a reasonable approximation for liquids like water, where large forces are required to compress the liquid or change the volume. In a swimming pool, for example, the density is approximately constant, and the water at the bottom is compressed very little by the weight of the water on top. Traveling up in the atmosphere is quite a different situation, however. The density of the air begins to change significantly just a short distance above Earth’s surface.

To derive a formula for the variation of pressure with depth in a tank containing a fluid of density ρ on the surface of Earth, we must start with the assumption that the density of the fluid is not constant. Fluid located at deeper levels is subjected to more force than fluid nearer to the surface due to the weight of the fluid above it. Therefore, the pressure calculated at a given depth is different than the pressure calculated using a constant density.