11.2 Angular momentum  (Page 4/8)

Angular momentum of a rigid body

We have investigated the angular momentum of a single particle, which we generalized to a system of particles. Now we can use the principles discussed in the previous section to develop the concept of the angular momentum of a rigid body. Celestial objects such as planets have angular momentum due to their spin and orbits around stars. In engineering, anything that rotates about an axis carries angular momentum, such as flywheels, propellers, and rotating parts in engines. Knowledge of the angular momenta of these objects is crucial to the design of the system in which they are a part.

To develop the angular momentum of a rigid body, we model a rigid body as being made up of small mass segments, $\text{Δ}{m}_i.$ In [link] , a rigid body is constrained to rotate about the z -axis with angular velocity $\omega$ . All mass segments that make up the rigid body undergo circular motion about the z -axis with the same angular velocity. Part (a) of the figure shows mass segment $\text{Δ}{m}_i$ with position vector ${\overrightarrow{r}}_i$ from the origin and radius $R_i$ to the z -axis. The magnitude of its tangential velocity is $v_i=R_i\omega$ . Because the vectors ${\overrightarrow{v}}_i\text{and}{\overrightarrow{r}}_i$ are perpendicular to each other, the magnitude of the angular momentum of this mass segment is

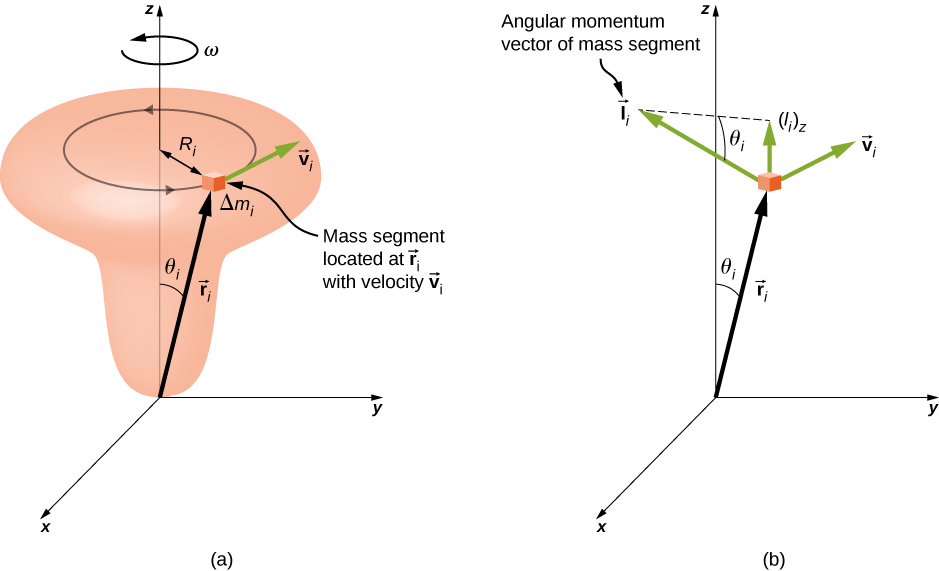
Using the right-hand rule, the angular momentum vector points in the direction shown in part (b). The sum of the angular momenta of all the mass segments contains components both along and perpendicular to the axis of rotation. Every mass segment has a perpendicular component of the angular momentum that will be cancelled by the perpendicular component of an identical mass segment on the opposite side of the rigid body. Thus, the component along the axis of rotation is the only component that gives a nonzero value when summed over all the mass segments. From part (b), the component of ${\overrightarrow{l}}_i$ along the axis of rotation is

The net angular momentum of the rigid body along the axis of rotation is

The summation ${{\displaystyle \sum _i\text{Δ}{m}_i(R_i)}}^2$ is simply the moment of inertia I of the rigid body about the axis of rotation. For a thin hoop rotating about an axis perpendicular to the plane of the hoop, all of the $R_i$ ’s are equal to R so the summation reduces to $R^2{\displaystyle \sum _i\text{Δ}{m}_i=m{R}^2},$ which is the moment of inertia for a thin hoop found in [link] . Thus, the magnitude of the angular momentum along the axis of rotation of a rigid body rotating with angular velocity $\omega$ about the axis is

This equation is analogous to the magnitude of the linear momentum $p=mv$ . The direction of the angular momentum vector is directed along the axis of rotation given by the right-hand rule.