10.8 Work and power for rotational motion  (Page 32/7)

Thus far in the chapter, we have extensively addressed kinematics and dynamics for rotating rigid bodies around a fixed axis. In this final section, we define work and power within the context of rotation about a fixed axis, which has applications to both physics and engineering. The discussion of work and power makes our treatment of rotational motion almost complete, with the exception of rolling motion and angular momentum, which are discussed in Angular Momentum . We begin this section with a treatment of the work-energy theorem for rotation.

Work for rotational motion

Now that we have determined how to calculate kinetic energy for rotating rigid bodies, we can proceed with a discussion of the work done on a rigid body rotating about a fixed axis. [link] shows a rigid body that has rotated through an angle $d\theta$ from A to B while under the influence of a force $\overrightarrow{F}$ . The external force $\overrightarrow{F}$ is applied to point P , whose position is $\overrightarrow{r}$ , and the rigid body is constrained to rotate about a fixed axis that is perpendicular to the page and passes through O . The rotational axis is fixed, so the vector $\overrightarrow{r}$ moves in a circle of radius r , and the vector $d\overrightarrow{s}$ is perpendicular to $\overrightarrow{r}.$

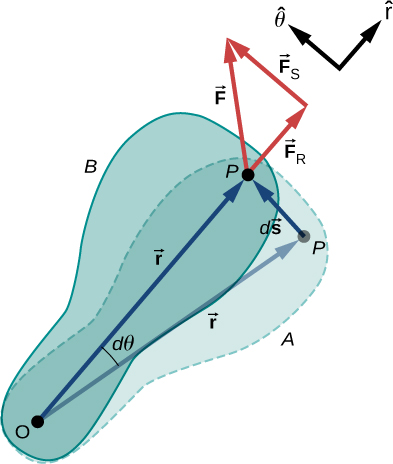
From [link] , we have

Thus,

Note that $d\overrightarrow{r}$ is zero because $\overrightarrow{r}$ is fixed on the rigid body from the origin O to point P . Using the definition of work, we obtain

where we used the identity $\overrightarrow{a}·(\overrightarrow{b}\times \overrightarrow{c})=\overrightarrow{b}·(\overrightarrow{c}\times \overrightarrow{a})$ . Noting that $(\overrightarrow{r}\times {\displaystyle \sum \overrightarrow{F}})={\displaystyle \sum \overrightarrow{\tau }}$ , we arrive at the expression for the rotational work    done on a rigid body:

The total work done on a rigid body is the sum of the torques integrated over the angle through which the body rotates . The incremental work is

where we have taken the dot product in [link] , leaving only torques along the axis of rotation. In a rigid body, all particles rotate through the same angle; thus the work of every external force is equal to the torque times the common incremental angle $d\theta$ . The quantity $\left({\displaystyle \sum _i{\tau }_i}\right)$ is the net torque on the body due to external forces.

Similarly, we found the kinetic energy of a rigid body rotating around a fixed axis by summing the kinetic energy of each particle that makes up the rigid body. Since the work-energy theorem $W_i=\text{Δ}{K}_i$ is valid for each particle, it is valid for the sum of the particles and the entire body.