| << Chapter < Page | Chapter >> Page > |
Many circuits also contain capacitors and inductors, in addition to resistors and an AC voltage source. We have seen how capacitors and inductors respond to DC voltage when it is switched on and off. We will now explore how inductors and capacitors react to sinusoidal AC voltage.
Suppose an inductor is connected directly to an AC voltage source, as shown in [link] . It is reasonable to assume negligible resistance, since in practice we can make the resistance of an inductor so small that it has a negligible effect on the circuit. Also shown is a graph of voltage and current as functions of time.
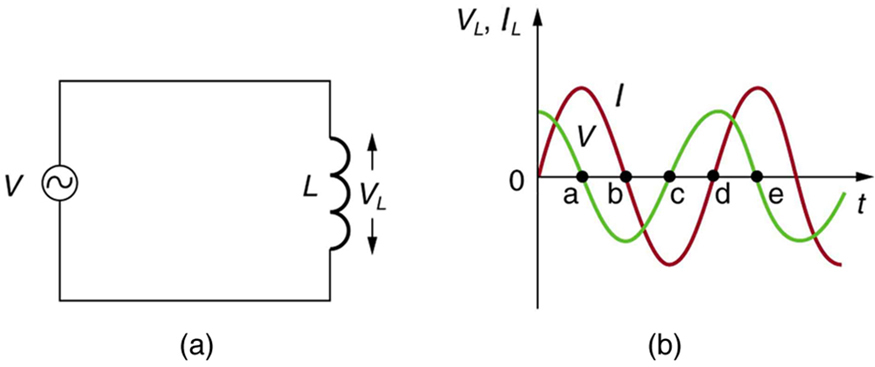
The graph in [link] (b) starts with voltage at a maximum. Note that the current starts at zero and rises to its peak after the voltage that drives it, just as was the case when DC voltage was switched on in the preceding section. When the voltage becomes negative at point a, the current begins to decrease; it becomes zero at point b, where voltage is its most negative. The current then becomes negative, again following the voltage. The voltage becomes positive at point c and begins to make the current less negative. At point d, the current goes through zero just as the voltage reaches its positive peak to start another cycle. This behavior is summarized as follows:
When a sinusoidal voltage is applied to an inductor, the voltage leads the current by one-fourth of a cycle, or by a phase angle.
Current lags behind voltage, since inductors oppose change in current. Changing current induces a back emf . This is considered to be an effective resistance of the inductor to AC. The rms current through an inductor is given by a version of Ohm’s law:
where is the rms voltage across the inductor and is defined to be
with the frequency of the AC voltage source in hertz (An analysis of the circuit using Kirchhoff’s loop rule and calculus actually produces this expression). is called the inductive reactance , because the inductor reacts to impede the current. has units of ohms ( , so that frequency times inductance has units of ), consistent with its role as an effective resistance. It makes sense that is proportional to , since the greater the induction the greater its resistance to change. It is also reasonable that is proportional to frequency , since greater frequency means greater change in current. That is, is large for large frequencies (large , small ). The greater the change, the greater the opposition of an inductor.
(a) Calculate the inductive reactance of a 3.00 mH inductor when 60.0 Hz and 10.0 kHz AC voltages are applied. (b) What is the rms current at each frequency if the applied rms voltage is 120 V?
Strategy
The inductive reactance is found directly from the expression . Once has been found at each frequency, Ohm’s law as stated in the Equation can be used to find the current at each frequency.
Solution for (a)
Entering the frequency and inductance into Equation gives
Similarly, at 10 kHz,
Solution for (b)
The rms current is now found using the version of Ohm’s law in Equation , given the applied rms voltage is 120 V. For the first frequency, this yields
Similarly, at 10 kHz,
Discussion
The inductor reacts very differently at the two different frequencies. At the higher frequency, its reactance is large and the current is small, consistent with how an inductor impedes rapid change. Thus high frequencies are impeded the most. Inductors can be used to filter out high frequencies; for example, a large inductor can be put in series with a sound reproduction system or in series with your home computer to reduce high-frequency sound output from your speakers or high-frequency power spikes into your computer.

Notification Switch
Would you like to follow the 'College physics' conversation and receive update notifications?