8.6 Analysis framework for laws of motion  (Page 11/3)

The analysis of motion involves writing Newton's second law of motion in mutually perpendicular coordinate directions. For this, we carry out force analysis following certain simple steps. These steps may appear too many, but we become experienced while working with them. Some of the steps are actually merged. Nevertheless, we need to recognize the importance of each of the steps as these are critical steps to ensure correct analysis of the motion/ situation. The steps are :

1. Identifying body system
2. Identifying external forces
3. Identifying a suitable coordinate system
4. Constructing a free-body diagram
5. Resolving force along the coordinate axes
6. Applying second law of motion

The identification of body system is based on the description of the problem. The body system decides the nature of force - whether it is internal or external. The selection of body system should suit the requirement of the analysis i.e. end objective of analysis. This will be clear as we discuss this aspect subsequently in the module.

The identification of external forces means to identify forces, which are applied on the object by other objects/sources and exclude forces, which the object applies on other objects. The important aspect of identification of external force is that external characterization depends on the definition of body system. The same force, which is external to a body, may become internal force for a different body system involving that body.

In this module, we shall not discuss steps 2 and 6. As far as identification of external forces (step 2) is concerned, we have discussed the same with an appropriate example in module titled Newton’s second law of motion . On the other hand, we shall apply second law of motion in mutually perpendicular directions (step 6) in the analysis of individual cases and as such we shall not discuss this step separately.

Identifying body system

If we are studying a single or two body system, then there is no issue. However, let us consider an illustration here as shown in the figure. There are possibilities of having different combination of bodies constituting different body systems.

Identifying body system

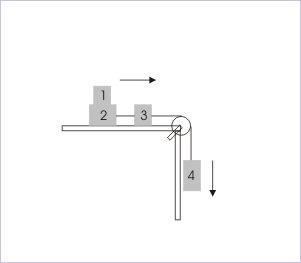
in selection of a body system, the guiding principle is to treat bodies as a single body system, when we know that bodies have common acceleration.

In the figure, the objects numbered 2 and 3 can be considered a single body system as two bodies have same acceleration. The objects 3 and 4 can not be combined as a single body system as they have acceleration of same magnitude, but with different direction. The objects 1 and 2 can be treated as a single body system, if two bodies do not have relative motion with respect to each other. Otherwise, we would need to treat them as separate body systems.

It is not always required that we must know the direction of acceleration before hand. We can assume a direction with respect to one body system and then proceed to find the directions of the accelerations of the other body systems in the arrangement. Even if our assumption about the direction of acceleration is incorrect, the solution of the problem automatically corrects the direction. We shall see this aspect while working with specially designed example, higlighting the issue.