| << Chapter < Page | Chapter >> Page > |
Questions and their answers are presented here in the module text format as if it were an extension of the treatment of the topic. The idea is to provide a verbose explanation, detailing the application of theory. Solution presented is, therefore, treated as the part of the understanding process – not merely a Q/A session. The emphasis is to enforce ideas and concepts, which can not be completely absorbed unless they are put to real time situation.
An online examination, based on the basic subject matter, is available at the link given here. The examination session is designed only for the basic level so that we can ensure that our understanding of subject matter is satisfactory.
Circular motion with constant acceleration
1: Visualize the circular motion as if we are dealing with straight line (pure translational) motion. Write down formula with substitution of linear quantities with angular quantities.
2: Use formula in scalar form. Stick to anticlockwise measurement as positive and clockwise measurement as negative.
We discuss problems, which highlight certain aspects of the study leading to circular motion with constant acceleration. The questions are categorized in terms of the characterizing features of the subject matter :
Problem : The angular velocity .vs. time plot of the motion of a rotating disk is shown in the figure.
Angular displacement .vs. time plot
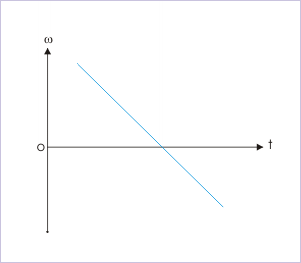
Determine (i) nature of angular velocity (ii) nature of angular acceleration and (iii) whether the disk comes to a standstill during the motion?
Solution : The angular velocity is anticlockwise (positive) above time axis and clockwise (negative) below time axis.
The slope of angular velocity - time plot indicates nature of acceleration. Since the plot is a straight line, motion is accelerated/ decelerated at constant rate. Further, the slope of the straight line (angular velocity - time plot) is negative all through out.
Recall that it is easy to determine the sign of the straight line. Just move from left to right in the direction of increasing time along the time - axis. See whether the angular velocity increases of decreases. If increases, then slope is positive; otherwise negative. The angular velocity, here, becomes less positive above time axis and becomes more negative below time axis. Hence, slope is negative all through out.
It means that acceleration (negative) is opposite to angular velocity (positive) above time axis. Therefore, the disk is decelerated and the angular speed of disk decreases at constant rate. Below time - axis, the angular acceleration is still negative. However, angular velocity is also negative below the time axis. As such, disk is accelerated and the angular speed increases at constant rate.
We see here that the plot intersects time - axis. It means that the disk comes to a stand still before changing direction from anticlockwise rotation to clockwise rotation.

Notification Switch
Would you like to follow the 'Physics for k-12' conversation and receive update notifications?