13.14 Conservation of energy (application)  (Page 21/2)

Questions and their answers are presented here in the module text format as if it were an extension of the treatment of the topic. The idea is to provide a verbose explanation, detailing the application of theory. Solution presented is, therefore, treated as the part of the understanding process – not merely a Q/A session. The emphasis is to enforce ideas and concepts, which can not be completely absorbed unless they are put to real time situation.

Representative problems and their solutions

We discuss problems, which highlight certain aspects of the study leading to the conservation of energy. The questions are categorized in terms of the characterizing features of the subject matter :

• Particle
• Projectile
• Pulley - block system
• Incline – block - spring system
• Vertical circular motion

Particle

Problem 1: The potential energy of a particle of mass "m" is given by " $\frac{1}{2}A{x}^2$ ". If non-conservative force is not involved and total mechanical energy is "E", then find its speed at "x".

Solution : External force and non-conservative force are absent here. Hence, we can apply conservation of mechanical energy. Total mechanical energy of the particle is constant,

$$E=U+K$$

$$\Rightarrow K=E-U$$

$$\Rightarrow K=E-\frac{1}{2}A{x}^2$$

$$\Rightarrow \frac{1}{2}m{v}^2=E-\frac{1}{2}A{x}^2$$

$$\Rightarrow v=\sqrt{\left\{\frac{\left(2E-A{x}^2\right)}{m}\right\}}$$

Projectile

Problem 2: A ball of 10 gm is fired from a spring gun of spring constant 500 N/m. The spring is initially compressed by 5 cm and is, then, released. Find the maximum horizontal distance (in meter) traveled by the bullet.

Solution : We are required to find maximum horizontal distance. The horizontal range of a projectile from the ground at an angle “θ” is given by :

Projectile

$$R=\frac{v^2\sin 2\theta }{g}$$

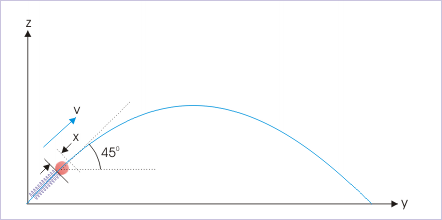
For maximum horizontal range, the angle of projection should be 45°. The maximum range is :

$$\Rightarrow R=\frac{v^2}{g}$$

In order to find speed of projection, we shall employ conservation of mechanical energy. The gun and ball forms an isolated system. Initially, kinetic energy of the bullet is zero, whereas initial elastic potential energy of the spring is “ $\frac{1}{2}k{x}^2$ ”. Let “v” be the speed when ball breaks the contact with spring. Now, applying conservation of mechanical energy,

$$K_i+U_i=K_f+U_f$$

Here,

$$K_i=0$$

$$U_i=\frac{1}{2}k{x}^2$$

$$K_f=\frac{1}{2}m{v}^2$$

$$U_f=0$$

Thus,

$$\Rightarrow \frac{1}{2}k{x}^2=\frac{1}{2}m{v}^2$$

$$\Rightarrow v^2=\frac{k{x}^2}{m}$$

Putting this expression in the expression of horizontal range, the maximum horizontal range is :

$$\Rightarrow R=\frac{k{x}^2}{mg}$$

$$\Rightarrow R=\frac{500X{\left(0.05\right)}^2}{0.01X10}=\frac{500x0.0025}{0.1}=\frac{1.25}{0.1}=12.5m$$

Pulley - block system

Problem 3: In the arrangement shown, the lighter block ascends a height of 2 m after being released. Find the speed of the blocks. Consider string to be mass-less and no friction at the contact.

Pulley - block system

Solution : Since friction is absent, only gravity interacts with the elements of the system. We can consider arrangement as isolated system. No external force acts on the system. Remember that Earth is part of the system and gravitational force is internal to the system. Hence, the situation fulfills all conditions for applying conservation of mechanical energy.

Now, two blocks are constrained by a taut string. It means that both blocks move with same speed. Let us denote 4 kg and 10 kg blocks with subscript “1” and “2”. Note that data is given for the change in height. Hence, it is easier to determine change in the potential energy.