| << Chapter < Page | Chapter >> Page > |
If a nuclide is known to decay, then its decay equation is
where Y is the nuclide having one more proton than X (see [link] ). So if you know that a certain nuclide decays, you can find the daughter nucleus by first looking up for the parent and then determining which element has atomic number . In the example of the decay of given earlier, we see that for Co and is Ni. It is as if one of the neutrons in the parent nucleus decays into a proton, electron, and neutrino. In fact, neutrons outside of nuclei do just that—they live only an average of a few minutes and decay in the following manner:
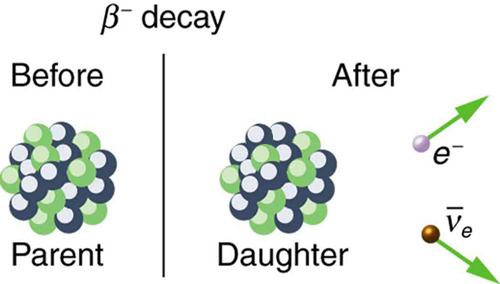
We see that charge is conserved in decay, since the total charge is before and after the decay. For example, in decay, total charge is 27 before decay, since cobalt has . After decay, the daughter nucleus is Ni, which has , and there is an electron, so that the total charge is also or 27. Angular momentum is conserved, but not obviously (you have to examine the spins and angular momenta of the final products in detail to verify this). Linear momentum is also conserved, again imparting most of the decay energy to the electron and the antineutrino, since they are of low and zero mass, respectively. Another new conservation law is obeyed here and elsewhere in nature. The total number of nucleons is conserved . In decay, for example, there are 60 nucleons before and after the decay. Note that total is also conserved in decay. Also note that the total number of protons changes, as does the total number of neutrons, so that total and total are not conserved in decay, as they are in decay. Energy released in decay can be calculated given the masses of the parent and products.
Find the energy emitted in the decay of .
Strategy and Concept
As in the preceding example, we must first find , the difference in mass between the parent nucleus and the products of the decay, using masses given in Appendix A . Then the emitted energy is calculated as before, using . The initial mass is just that of the parent nucleus, and the final mass is that of the daughter nucleus and the electron created in the decay. The neutrino is massless, or nearly so. However, since the masses given in Appendix A are for neutral atoms, the daughter nucleus has one more electron than the parent, and so the extra electron mass that corresponds to the is included in the atomic mass of Ni. Thus,
Solution
The decay equation for is
As noticed,
Entering the masses found in Appendix A gives
Thus,
Using , we obtain
Discussion and Implications
Perhaps the most difficult thing about this example is convincing yourself that the mass is included in the atomic mass of . Beyond that are other implications. Again the decay energy is in the MeV range. This energy is shared by all of the products of the decay. In many decays, the daughter nucleus is left in an excited state and emits photons ( rays). Most of the remaining energy goes to the electron and neutrino, since the recoil kinetic energy of the daughter nucleus is small. One final note: the electron emitted in decay is created in the nucleus at the time of decay.

Notification Switch
Would you like to follow the 'College physics' conversation and receive update notifications?