Friction  (Page 2/12)

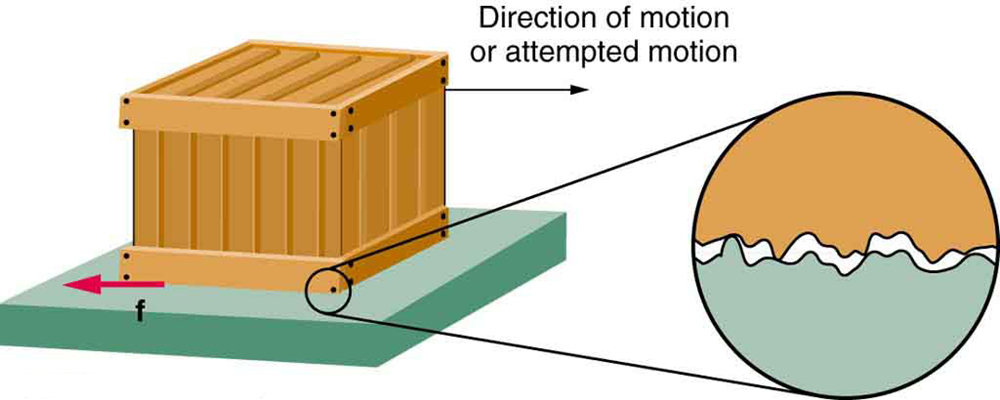
The magnitude of the frictional force has two forms: one for static situations (static friction), the other for when there is motion (kinetic friction).

When there is no motion between the objects, the magnitude of static friction ${\mathbf{f}}_{\text{s}}$ is

where ${\mu }_{\text{s}}$ is the coefficient of static friction and $N$ is the magnitude of the normal force (the force perpendicular to the surface).

The symbol means less than or equal to , implying that static friction can have a minimum and a maximum value of ${\mu }_{\text{s}}N$ . Static friction is a responsive force that increases to be equal and opposite to whatever force is exerted, up to its maximum limit. Once the applied force exceeds $f_{\text{s}(\text{max})}$ , the object will move. Thus

Once an object is moving, the magnitude of kinetic friction ${\mathbf{f}}_{\text{k}}$ is given by

where ${\mu }_{\text{k}}$ is the coefficient of kinetic friction. A system in which $f_{\text{k}}={\mu }_{\text{k}}N$ is described as a system in which friction behaves simply .

As seen in [link] , the coefficients of kinetic friction are less than their static counterparts. That values of $\mu$ in [link] are stated to only one or, at most, two digits is an indication of the approximate description of friction given by the above two equations.

The equations given earlier include the dependence of friction on materials and the normal force. The direction of friction is always opposite that of motion, parallel to the surface between objects, and perpendicular to the normal force. For example, if the crate you try to push (with a force parallel to the floor) has a mass of 100 kg, then the normal force would be equal to its weight, $W=\mathrm{mg}=(\text{100 kg})(9\text{.}\text{80}{\text{m/s}}^2)=\text{980 N}$ , perpendicular to the floor. If the coefficient of static friction is 0.45, you would have to exert a force parallel to the floor greater than $f_{\text{s}(\text{max})}={\mu }_{\text{s}}N=\left(0.45\right)(\text{980}\text{N})=\text{440}\text{N}$ to move the crate. Once there is motion, friction is less and the coefficient of kinetic friction might be 0.30, so that a force of only 290 N( $f_{\text{k}}={\mu }_{\text{k}}N=\left(0\text{.}\text{30}\right)\left(\text{980}\text{N}\right)=\text{290}\text{N}$ ) would keep it moving at a constant speed. If the floor is lubricated, both coefficients are considerably less than they would be without lubrication. Coefficient of friction is a unit less quantity with a magnitude usually between 0 and 1.0. The coefficient of the friction depends on the two surfaces that are in contact.