9.3 Comparing two independent population proportions

1. The two independent samples are simple random samples that are independent.
2. The number of successes is at least five and the number of failures is at least five for each of the samples.

Comparing two proportions, like comparing two means, is common. If two estimated proportions are different, it may be due to a difference in the populationsor it may be due to chance. A hypothesis test can help determine if a difference in the estimated proportions $(P_A-P_B)$ reflects a difference in the population proportions.

The difference of two proportions follows an approximate normal distribution. Generally, the null hypothesis states that the two proportions are the same. That is, $H_o:p_A=p_B$ . To conduct the test, we use a pooled proportion, $p_c$ .

Two population proportions

Two types of medication for hives are being tested to determine if there is a difference in the proportions of adult patient reactions. Twenty out of a random sample of 200 adults given medication A still had hives 30 minutes after taking the medication. Twelve out of another random sample of 200 adults given medication B still had hives 30 minutes after taking the medication. Test at a 1% level of significance.

Determining the solution

This is a test of 2 population proportions.

Let $A$ and $B$ be the subscripts for medication A and medication B. Then $p_A$ and $p_B$ are the desired population proportions.

Random variable:

$H_o:p_A=p_B{p}_A-p_B=0$

$H_a:p_A\ne p_B{p}_A-p_B\ne 0$

The words "is a difference" tell you the test is two-tailed.

Distribution for the test: Since this is a test of two binomial population proportions, the distribution is normal:

$p_c=\frac{x_A+x_B}{n_A+n_B}=\frac{20+12}{200+200}=0.081-p_c=0.92$

Therefore, ${\mathrm{P\text{'}}}_A-{\mathrm{P\text{'}}}_B~N[0,\sqrt{\left(0.08\right)\cdot \left(0.92\right)\cdot (\frac{1}{200}+\frac{1}{200})}]$

${\mathrm{P\text{'}}}_A-{\mathrm{P\text{'}}}_B$ follows an approximate normal distribution.

Calculate the p-value using the normal distribution: p-value = 0.1404.

Estimated proportion for group A: ${\mathrm{p\text{'}}}_A=\frac{x_A}{n_A}=\frac{20}{200}=0.1$

Estimated proportion for group B: ${\mathrm{p\text{'}}}_B=\frac{x_B}{n_B}=\frac{12}{200}=0.06$

Graph:

${\mathrm{P\text{'}}}_A-{\mathrm{P\text{'}}}_B=0.1-0.06=0.04$ .

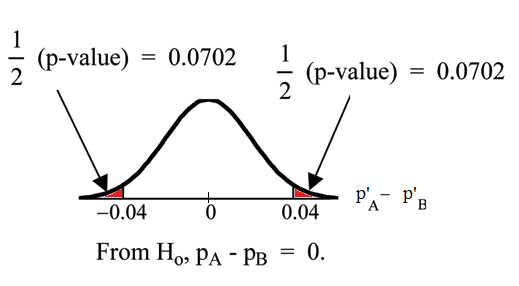
Half the p-value is below -0.04 andhalf is above 0.04.

Compare $\alpha$ and the p-value: $\alpha =0.01$ and the $\text{p-value}=0.1404$ . $\alpha <$ p-value.

Make a decision: Since $\alpha <\text{p-value}$ , do not reject $H_o$ .

Conclusion: At a 1% level of significance, from the sample data, there is not sufficient evidence to conclude that there is a difference in the proportions of adultpatients who did not react after 30 minutes to medication A and medication B.