8.3 Resistance and resistivity  (Page 3/6)

The resistance of an object also depends on temperature, since $R_0$ is directly proportional to $\rho$ . For a cylinder we know $R=\mathrm{\rho L}/A$ , and so, if $L$ and $A$ do not change greatly with temperature, $R$ will have the same temperature dependence as $\rho$ . (Examination of the coefficients of linear expansion shows them to be about two orders of magnitude less than typical temperature coefficients of resistivity, and so the effect of temperature on $L$ and $A$ is about two orders of magnitude less than on $\rho$ .) Thus,

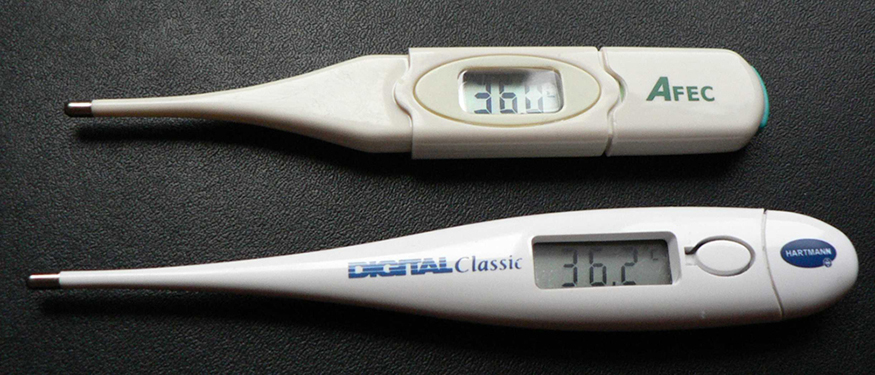
is the temperature dependence of the resistance of an object, where $R_0$ is the original resistance and $R$ is the resistance after a temperature change $\mathrm{\Delta }T$ . Numerous thermometers are based on the effect of temperature on resistance. (See [link] .) One of the most common is the thermistor, a semiconductor crystal with a strong temperature dependence, the resistance of which is measured to obtain its temperature. The device is small, so that it quickly comes into thermal equilibrium with the part of a person it touches.

Phet explorations: resistance in a wire

Learn about the physics of resistance in a wire. Change its resistivity, length, and area to see how they affect the wire's resistance. The sizes of the symbols in the equation change along with the diagram of a wire.

Section summary

• The resistance $R$ of a cylinder of length $L$ and cross-sectional area $A$ is $R=\frac{\mathrm{\rho L}}{A}$ , where $\rho$ is the resistivity of the material.
• Values of $\rho$ in [link] show that materials fall into three groups— conductors, semiconductors, and insulators .
• Temperature affects resistivity; for relatively small temperature changes $\mathrm{\Delta }T$ , resistivity is $\rho ={\rho }_0(\text{1}+\alpha \mathrm{\Delta }T)$ , where ${\rho }_0$ is the original resistivity and $\text{α}$ is the temperature coefficient of resistivity.
• [link] gives values for $\alpha$ , the temperature coefficient of resistivity.
• The resistance $R$ of an object also varies with temperature: $R=R_0(\text{1}+\alpha \mathrm{\Delta }T)$ , where $R_0$ is the original resistance, and $R$ is the resistance after the temperature change.

Conceptual questions