8.10 Examples (Page 4/6)

Collaborative statistics (custom Page 4 / 6

Suppose a consumer group suspects that the proportion of households that have three cell phones is 30%. A cell phone company has reason to believe that the proportion is 30%. Before they start a big advertising campaign, they conduct a hypothesis test. Their marketing people survey 150 households with theresult that 43 of the households have three cell phones.

Set up the Hypothesis Test:

$H_{o}$ : $p$ $= 0.30$ $H_{a}$ : $p$ $0.30$

Determine the distribution needed:

The random variable is $P'$ = proportion of households that have three cell phones.

The distribution for the hypothesis test is $P'$ ~ $N$ $(0.30, \sqrt{\frac{(0.30) \cdot (0.70)}{150}})$

The value that helps determine the p-value is $p'$ . Calculate $p'$ .

$p' = \frac{x}{n}$ where $x$ is the number of successes and $n$ is the total number in the sample.

$x = 43$ , $n = 150$

p' = $\frac{43}{150}$

What is a success for this problem?

A success is having three cell phones in a household.

What is the level of significance?

The level of significance is the preset $α$ . Since $α$ is not given, assume that $α = 0.05$ .

Draw the graph for this problem. Draw the horizontal axis. Label and shade appropriately.

Calculate the p-value.

p-value = 0.7216

Make a decision. _____________(Reject/Do not reject) $H_{0}$ because____________.

Assuming that $α$ = 0.05, $α < p-value$ . The Decision is do not reject $H_{0}$ because there is not sufficient evidence to conclude that the proportion of households that have three cell phones is not 30%.

The next example is a poem written by a statistics student named Nicole Hart. The solution to the problem follows the poem. Notice that the hypothesis test is for a singlepopulation proportion. This means that the null and alternate hypotheses use theparameter $p$ . The distribution for the test is normal. The estimated proportion $p'$ is the proportion of fleas killed to the total fleas found on Fido. This is sample information.The problem gives a preconceived $α = 0.01$ , for comparison, and a 95% confidence interval computation. The poem is clever and humorous, so please enjoy it!

Hypothesis testing problems consist of multiple steps. To help you do the problems, solution sheets are provided for your use. Look in the Table of Contents Appendix for the topic "Solution Sheets." If you like, use copies of the appropriate solution sheet for homework problems.

My dog has so many fleas, They do not come off with ease.As for shampoo, I have tried many types Even one called Bubble Hype,Which only killed 25% of the fleas, Unfortunately I was not pleased.I've used all kinds of soap, Until I had give up hopeUntil one day I saw An ad that put me in awe.A shampoo used for dogs Called GOOD ENOUGH to Clean a HogGuaranteed to kill more fleas. I gave Fido a bathAnd after doing the math His number of fleasStarted dropping by 3's! Before his shampooI counted 42. At the end of his bath,I redid the math And the new shampoo had killed 17 fleas.So now I was pleased. Now it is time for you to have some funWith the level of significance being .01, You must help me figure outUse the new shampoo or go without?

Set up the Hypothesis Test:

$H_{o}$ : $p = 0.25$ $H_{a}$ : $p > 0.25$

Determine the distribution needed:

In words, CLEARLY state what your random variable $X$ or $P'$ represents.

$P'$ = The proportion of fleas that are killed by the new shampoo

State the distribution to use for the test.

Normal: $N (0.25, \sqrt{\frac{(0.25) (1 - 0.25)}{42}})$

Test Statistic: $z = 2.3163$

Calculate the p-value using the normal distribution for proportions:

$p-value =$ $0.0103$

In 1 – 2 complete sentences, explain what the p-value means for this problem.

If the null hypothesis is true (the proportion is 0.25), then there is a 0.0103 probability that the sample (estimated) proportion is 0.4048 $(\frac{17}{42})$ or more.

Use the previous information to sketch a picture of this situation. CLEARLY, label and scale the horizontal axis and shade the region(s) corresponding to the p-value.

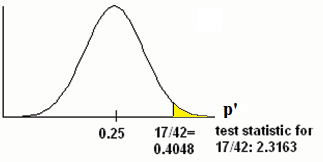
Normal distribution graph of the proportion of fleas killed by the new shampoo with values of 0.25 and 0.4048 on the x-axis. A vertical upward line extends from 0.4048 to the curve and the area to the left of this is shaded in. The test statistic of the sample proportion is listed.

Compare $α$ and the p-value:

Indicate the correct decision (“reject” or “do not reject” the null hypothesis), the reason for it, and write an appropriate conclusion, using COMPLETE SENTENCES.

alpha	decision	reason for decision
0.01	Do not reject $H_{o}$	$α$ $p-value$

Conclusion: At the 1% level of significance, the sample data do not show sufficient evidence that the percentage of fleas that are killed by the new shampoo is more than 25%.

Construct a 95% Confidence Interval for the true mean or proportion. Include a sketch of the graph of the situation. Label the point estimate and the lower and upper bounds of the Confidence Interval.

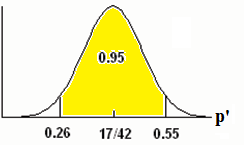
Normal distribution graph of the proportion of fleas killed by the new shampoo with values of 0.26, 17/42, and 0.55 on the x-axis. A vertical upward line extends from 0.26 and 0.55. The area between these two points is equal to 0.95.

Confidence Interval: $(0.26, 0.55)$ We are 95% confident that the true population proportion $p$ of fleas that are killed by the new shampoo is between 26% and 55%.

This test result is not very definitive since the p-value is very close to alpha. In reality, one would probably do more tests by giving the dog another bath after the fleas have had a chance to return.

<< Chapter < Page Page > Chapter >>

Practice Key Terms 2

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, Collaborative statistics (custom lecture version modified by t. short). OpenStax CNX. Jul 15, 2013 Download for free at http://cnx.org/content/col11543/1.1

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Collaborative statistics (custom lecture version modified by t. short)' conversation and receive update notifications?

Ask

	13 Biology 13 Modern Understandings of Inheritance By OpenStax Start Quiz
	How well do you know 5SOS? By Wey Hey Start Quiz
©flickr: Justin	Music Appreciation Final Practice By Madison Christian Start Exam
	NCE Ch 05 Theories in Counseling Helping... By Anh Dao Start Exam
	30 Biology 30 Plant Form and Physiology MCQ By OpenStax Start Quiz
©flickr: U.S.	Molecular Cellular Biology By Ann Schlosser Start Quiz
	Are you a 5sos fan? By Rylee Minllic Start Quiz
©flickr:	Word Roots and Prefixes By Ellie Banfield Start Quiz
	Cultural Anthropology Assignment 2 By Richley Crapo Start Assignment
	Vocabulary Week 1-3 By Rachel Woolard Start Quiz