| << Chapter < Page | Chapter >> Page > |
Very large forces are produced in joints when a person jumps from some height to the ground. (a) Calculate the magnitude of the force produced if an 80.0-kg person jumps from a 0.600–m-high ledge and lands stiffly, compressing joint material 1.50 cm as a result. (Be certain to include the weight of the person.) (b) In practice the knees bend almost involuntarily to help extend the distance over which you stop. Calculate the magnitude of the force produced if the stopping distance is 0.300 m. (c) Compare both forces with the weight of the person.
(a)
(b)
(c) Ratio of net force to weight of person is 41.0 in part (a); 3.00 in part (b)
Jogging on hard surfaces with insufficiently padded shoes produces large forces in the feet and legs. (a) Calculate the magnitude of the force needed to stop the downward motion of a jogger’s leg, if his leg has a mass of 13.0 kg, a speed of 6.00 m/s, and stops in a distance of 1.50 cm. (Be certain to include the weight of the 75.0-kg jogger’s body.) (b) Compare this force with the weight of the jogger.
(a) Calculate the energy in kJ used by a 55.0-kg woman who does 50 deep knee bends in which her center of mass is lowered and raised 0.400 m. (She does work in both directions.) You may assume her efficiency is 20%. (b) What is the average power consumption rate in watts if she does this in 3.00 min?
(a) 108 kJ
(b) 599 W
Kanellos Kanellopoulos flew 119 km from Crete to Santorini, Greece, on April 23, 1988, in the Daedalus 88 , an aircraft powered by a bicycle-type drive mechanism (see [link] ). His useful power output for the 234-min trip was about 350 W. Using the efficiency for cycling from [link] , calculate the food energy in kilojoules he metabolized during the flight.

The swimmer shown in [link] exerts an average horizontal backward force of 80.0 N with his arm during each 1.80 m long stroke. (a) What is his work output in each stroke? (b) Calculate the power output of his arms if he does 120 strokes per minute.
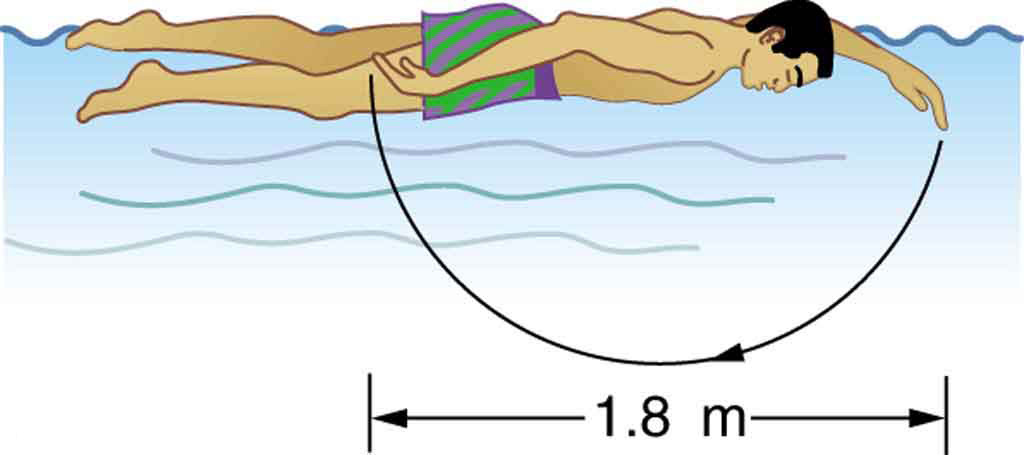
(a) 144 J
(b) 288 W
Mountain climbers carry bottled oxygen when at very high altitudes. (a) Assuming that a mountain climber uses oxygen at twice the rate for climbing 116 stairs per minute (because of low air temperature and winds), calculate how many liters of oxygen a climber would need for 10.0 h of climbing. (These are liters at sea level.) Note that only 40% of the inhaled oxygen is utilized; the rest is exhaled. (b) How much useful work does the climber do if he and his equipment have a mass of 90.0 kg and he gains 1000 m of altitude? (c) What is his efficiency for the 10.0-h climb?
The awe-inspiring Great Pyramid of Cheops was built more than 4500 years ago. Its square base, originally 230 m on a side, covered 13.1 acres, and it was 146 m high, with a mass of about . (The pyramid’s dimensions are slightly different today due to quarrying and some sagging.) Historians estimate that 20,000 workers spent 20 years to construct it, working 12-hour days, 330 days per year. (a) Calculate the gravitational potential energy stored in the pyramid, given its center of mass is at one-fourth its height. (b) Only a fraction of the workers lifted blocks; most were involved in support services such as building ramps (see [link] ), bringing food and water, and hauling blocks to the site. Calculate the efficiency of the workers who did the lifting, assuming there were 1000 of them and they consumed food energy at the rate of 300 kcal/h. What does your answer imply about how much of their work went into block-lifting, versus how much work went into friction and lifting and lowering their own bodies? (c) Calculate the mass of food that had to be supplied each day, assuming that the average worker required 3600 kcal per day and that their diet was 5% protein, 60% carbohydrate, and 35% fat. (These proportions neglect the mass of bulk and nondigestible materials consumed.)
(a)
(b) 2.52%
(c) (14 metric tons)
(a) How long can you play tennis on the 800 kJ (about 200 kcal) of energy in a candy bar? (b) Does this seem like a long time? Discuss why exercise is necessary but may not be sufficient to cause a person to lose weight.

Notification Switch
Would you like to follow the 'Physics 110 at une' conversation and receive update notifications?