7.2 Elastic energy  (Page 3/8)

Using conservation of mechanical energy to calculate the speed of a toy car

A 0.100-kg toy car is propelled by a compressed spring, as shown in [link] . The car follows a track that rises 0.180 m above the starting point. The spring is compressed 4.00 cm and has a force constant of 250.0 N/m. Assuming work done by friction to be negligible, find (a) how fast the car is going before it starts up the slope and (b) how fast it is going at the top of the slope.

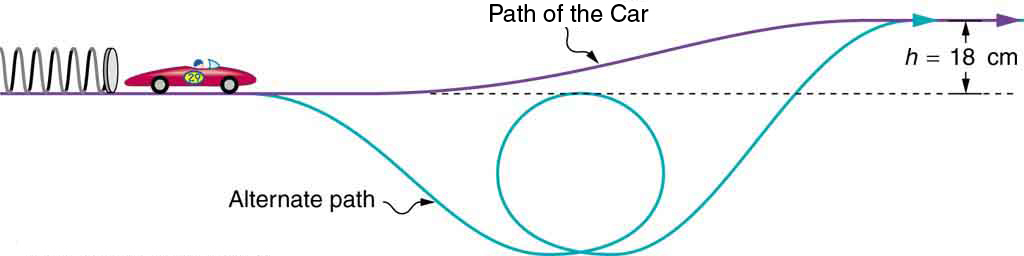
Strategy

The spring force and the gravitational force are conservative forces, so conservation of mechanical energy can be used. Thus,

or

where $h$ is the height (vertical position) and $x$ is the compression of the spring. This general statement looks complex but becomes much simpler when we start considering specific situations. First, we must identify the initial and final conditions in a problem; then, we enter them into the last equation to solve for an unknown.

Solution for (a)

This part of the problem is limited to conditions just before the car is released and just after it leaves the spring. Take the initial height to be zero, so that both $h_{\text{i}}$ and $h_{\text{f}}$ are zero. Furthermore, the initial speed $v_{\text{i}}$ is zero and the final compression of the spring $x_{\text{f}}$ is zero, and so several terms in the conservation of mechanical energy equation are zero and it simplifies to

In other words, the initial potential energy in the spring is converted completely to kinetic energy in the absence of friction. Solving for the final speed and entering known values yields

Solution for (b)

One method of finding the speed at the top of the slope is to consider conditions just before the car is released and just after it reaches the top of the slope, completely ignoring everything in between. Doing the same type of analysis to find which terms are zero, the conservation of mechanical energy becomes

This form of the equation means that the spring’s initial potential energy is converted partly to gravitational potential energy and partly to kinetic energy. The final speed at the top of the slope will be less than at the bottom. Solving for $v_{\text{f}}$ and substituting known values gives

Discussion

Another way to solve this problem is to realize that the car’s kinetic energy before it goes up the slope is converted partly to potential energy—that is, to take the final conditions in part (a) to be the initial conditions in part (b).

Note that, for conservative forces, we do not directly calculate the work they do; rather, we consider their effects through their corresponding potential energies, just as we did in [link] . Note also that we do not consider details of the path taken—only the starting and ending points are important (as long as the path is not impossible). This assumption is usually a tremendous simplification, because the path may be complicated and forces may vary along the way.

Phet explorations: energy skate park

Learn about conservation of energy with a skater dude! Build tracks, ramps and jumps for the skater and view the kinetic energy, potential energy and friction as he moves. You can also take the skater to different planets or even space!

Section summary

• A conservative force is one for which work depends only on the starting and ending points of a motion, not on the path taken.
• We can define potential energy $(\text{PE})$ for any conservative force, just as we defined ${\text{PE}}_{\mathrm{g}}$ for the gravitational force.
• The potential energy of a spring is ${\text{PE}}_{\mathrm{s}}=\frac{1}{2}{\text{kx}}^2$ , where $k$ is the spring’s force constant and $x$ is the displacement from its undeformed position.
• Mechanical energy is defined to be $\text{KE}+\text{PE}$ for a conservative force.
• When only conservative forces act on and within a system, the total mechanical energy is constant. In equation form,

where i and f denote initial and final values. This is known as the conservation of mechanical energy.

Conceptual questions

Problems&Exercises