6.6 Conservation of energy  (Page 3/10)

Another example of energy conversion occurs in a solar cell. Sunlight impinging on a solar cell (see [link] ) produces electricity, which in turn can be used to run an electric motor. Energy is converted from the primary source of solar energy into electrical energy and then into mechanical energy.

Efficiency

Even though energy is conserved in an energy conversion process, the output of useful energy or work will be less than the energy input. The efficiency     $\text{Eff}$ of an energy conversion process is defined as

[link] lists some efficiencies of mechanical devices and human activities. In a coal-fired power plant, for example, about 40% of the chemical energy in the coal becomes useful electrical energy. The other 60% transforms into other (perhaps less useful) energy forms, such as thermal energy, which is then released to the environment through combustion gases and cooling towers.

Phet explorations: masses and springs

A realistic mass and spring laboratory. Hang masses from springs and adjust the spring stiffness and damping. You can even slow time. Transport the lab to different planets. A chart shows the kinetic, potential, and thermal energies for each spring.

Section summary

• The law of conservation of energy states that the total energy is constant in any process. Energy may change in form or be transferred from one system to another, but the total remains the same.
• When all forms of energy are considered, conservation of energy is written in equation form as ${\text{KE}}_{\mathrm{i}}+{\text{PE}}_{\mathrm{i}}+W_{\text{nc}}+{\text{OE}}_{\mathrm{i}}={\text{KE}}_{\mathrm{f}}+{\text{PE}}_{\mathrm{f}}+{\text{OE}}_{\mathrm{f}}$ , where $\text{OE}$ is all other forms of energy besides mechanical energy.
• Commonly encountered forms of energy include electric energy, chemical energy, radiant energy, nuclear energy, and thermal energy.
• Energy is often utilized to do work, but it is not possible to convert all the energy of a system to work.
• The efficiency $\text{Eff}$ of a machine or human is defined to be $\text{Eff}=\frac{W_{\text{out}}}{E_{\text{in}}}$ , where $W_{\text{out}}$ is useful work output and $E_{\text{in}}$ is the energy consumed.

Conceptual questions

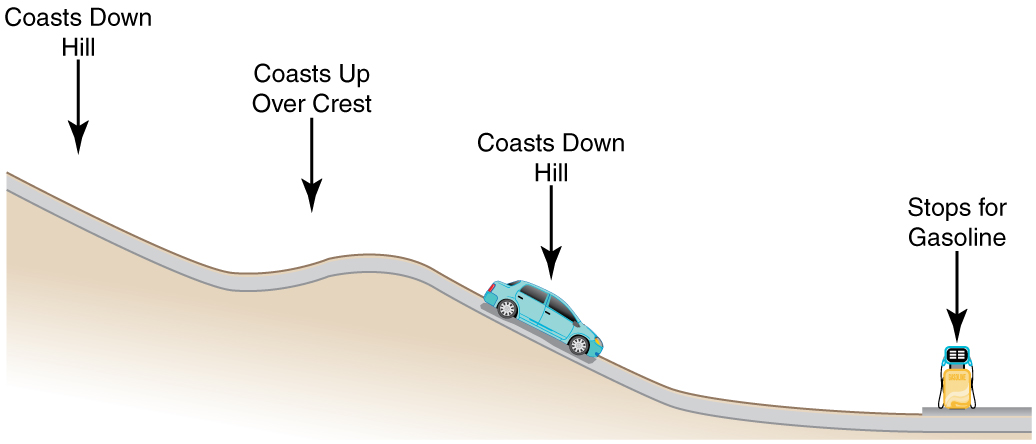
Consider the following scenario. A car for which friction is not negligible accelerates from rest down a hill, running out of gasoline after a short distance. The driver lets the car coast farther down the hill, then up and over a small crest. He then coasts down that hill into a gas station, where he brakes to a stop and fills the tank with gasoline. Identify the forms of energy the car has, and how they are changed and transferred in this series of events. (See [link] .)

Problems&Exercises