1. Home
  2. Physics subject knowledge
  3. 5. geometric optics
  4. Total internal reflection

6.4 Total internal reflection  (Page 9/8)

<< Chapter < Page
  Physics subject knowledge     Page 1 / 8
Chapter >> Page >
  • Explain the phenomenon of total internal reflection.
  • Describe the workings and uses of fiber optics.
  • Analyze the reason for the sparkle of diamonds.

A good-quality mirror may reflect more than 90% of the light that falls on it, absorbing the rest. But it would be useful to have a mirror that reflects all of the light that falls on it. Interestingly, we can produce total reflection using an aspect of refraction .

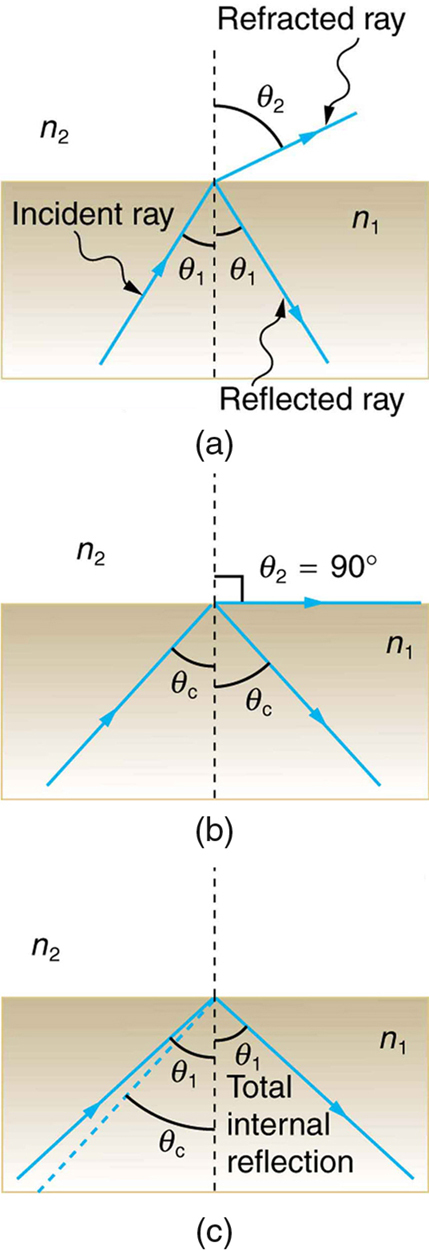
Consider what happens when a ray of light strikes the surface between two materials, such as is shown in [link] (a). Part of the light crosses the boundary and is refracted; the rest is reflected. If, as shown in the figure, the index of refraction for the second medium is less than for the first, the ray bends away from the perpendicular. (Since n 1 > n 2 size 12{n rSub { size 8{1} }>n rSub { size 8{2} } } {} , the angle of refraction is greater than the angle of incidence—that is, θ 1 > θ 2 size 12{θ rSub { size 8{1} }>θ rSub { size 8{2} } } {} .) Now imagine what happens as the incident angle is increased. This causes θ 2 size 12{θ rSub { size 8{2} } } {} to increase also. The largest the angle of refraction θ 2 size 12{θ rSub { size 8{2} } } {} can be is 90º size 12{"90"°} {} , as shown in [link] (b).The critical angle     θ c size 12{θ rSub { size 8{c} } } {} for a combination of materials is defined to be the incident angle θ 1 size 12{θ rSub { size 8{1} } } {} that produces an angle of refraction of 90º . That is, θ c is the incident angle for which θ 2 = 90º . If the incident angle θ 1 is greater than the critical angle, as shown in [link] (c), then all of the light is reflected back into medium 1, a condition called total internal reflection .

Critical angle

The incident angle θ 1 size 12{θ rSub { size 8{1} } } {} that produces an angle of refraction of 90º size 12{"90"°} {} is called the critical angle, θ c size 12{θ rSub { size 8{1} } } {} .

In the first figure, an incident ray at an angle theta 1 with a perpendicular line drawn at the point of incidence travels from n1 to n2. The incident ray suffers both refraction and reflection. The angle of refraction is theta 2. In the second figure, as theta 1 is increased, the angle of refraction theta 2 becomes 90 degrees and the angle of reflection corresponding to 90 degrees is theta c. In the third figure, theta c greater than theta i, total internal reflection takes place and instead of refraction, reflection takes place and the light ray travels back into medium n1.
(a) A ray of light crosses a boundary where the speed of light increases and the index of refraction decreases. That is, n 2 < n 1 size 12{n rSub { size 8{2} }<n rSub { size 8{1} } } {} . The ray bends away from the perpendicular. (b) The critical angle θ c size 12{θ rSub { size 8{c} } } {} is the one for which the angle of refraction is . (c) Total internal reflection occurs when the incident angle is greater than the critical angle.

Snell’s law states the relationship between angles and indices of refraction. It is given by

n 1 sin θ 1 = n 2 sin θ 2 . size 12{n rSub { size 8{1} } "sin"θ rSub { size 8{1} } =n rSub { size 8{2} } "sin"θ rSub { size 8{2} } } {}

When the incident angle equals the critical angle ( θ 1 = θ c size 12{θ rSub { size 8{1} } =`θ rSub { size 8{c} } } {} ), the angle of refraction is 90º size 12{"90"°} {} ( θ 2 = 90º size 12{θ rSub { size 8{2} } =`"90" rSup { size 8{0} } } {} ). Noting that sin 90º =1 size 12{"sin"" 90"°"=1"} {} , Snell’s law in this case becomes

n 1 sin θ 1 = n 2 . size 12{n rSub { size 8{1} } "sin"θ rSub { size 8{1} } =n rSub { size 8{2} } } {}

The critical angle θ c size 12{q rSub { size 8{c} } } {} for a given combination of materials is thus

θ c = sin − 1 n 2 / n 1 for size 12{θ rSub { size 8{c} } ="sin" rSup { size 8{ - 1} } left ( {n rSub { size 8{2} } } slash {n rSub { size 8{1} } } right )} {} n 1 > n 2 . size 12{n rSub { size 8{1} }>n rSub { size 8{2} } } {}

Total internal reflection occurs for any incident angle greater than the critical angle θ c size 12{q rSub { size 8{c} } } {} , and it can only occur when the second medium has an index of refraction less than the first. Note the above equation is written for a light ray that travels in medium 1 and reflects from medium 2, as shown in the figure.

How big is the critical angle here?

What is the critical angle for light traveling in a polystyrene (a type of plastic) pipe surrounded by air?

Strategy

The index of refraction for polystyrene is found to be 1.49 in [link] , and the index of refraction of air can be taken to be 1.00, as before. Thus, the condition that the second medium (air) has an index of refraction less than the first (plastic) is satisfied, and the equation θ c = sin − 1 n 2 / n 1 size 12{θ rSub { size 8{c} } ="sin" rSup { size 8{ - 1} } left ( {n rSub { size 8{2} } } slash {n rSub { size 8{1} } } right )} {} can be used to find the critical angle θ c size 12{θ rSub { size 8{c} } } {} . Here, then, n 2 = 1 . 00 size 12{n rSub { size 8{2} } =1 "." "00"} {} and n 1 = 1 . 49 size 12{n rSub { size 8{1} } =1 "." "49"} {} .

Solution

The critical angle is given by

θ c = sin − 1 n 2 / n 1 . size 12{θ rSub { size 8{c} } ="sin" rSup { size 8{ - 1} } left ( {n rSub { size 8{2} } } slash {n rSub { size 8{1} } } right )} {}

Substituting the identified values gives

θ c = sin − 1 1 . 00 / 1 . 49 = sin − 1 0 . 671 42.2º. alignl { stack { size 12{θ rSub { size 8{c} } ="sin" rSup { size 8{ - 1} } left ( {1 "." "00"} slash {1 "." "49"} right )="sin" rSup { size 8{ - 1} } left (0 "." "671" right )} {} #="42" "." 2° "." {} } } {}

Discussion

This means that any ray of light inside the plastic that strikes the surface at an angle greater than 42.2º will be totally reflected. This will make the inside surface of the clear plastic a perfect mirror for such rays without any need for the silvering used on common mirrors. Different combinations of materials have different critical angles, but any combination with n 1 > n 2 size 12{n rSub { size 8{1} }>n rSub { size 8{2} } } {} can produce total internal reflection. The same calculation as made here shows that the critical angle for a ray going from water to air is 48 . 6º size 12{"48" "." 6°} {} , while that from diamond to air is 24 . 4º size 12{"24" "." 4°} {} , and that from flint glass to crown glass is 66 . 3º size 12{"66" "." 3°} {} . There is no total reflection for rays going in the other direction—for example, from air to water—since the condition that the second medium must have a smaller index of refraction is not satisfied. A number of interesting applications of total internal reflection follow.

Questions & Answers

A golfer on a fairway is 70 m away from the green, which sits below the level of the fairway by 20 m. If the golfer hits the ball at an angle of 40° with an initial speed of 20 m/s, how close to the green does she come?
Aislinn Reply
cm
tijani
what is titration
John Reply
what is physics
Siyaka Reply
A mouse of mass 200 g falls 100 m down a vertical mine shaft and lands at the bottom with a speed of 8.0 m/s. During its fall, how much work is done on the mouse by air resistance
Jude Reply
Can you compute that for me. Ty
Jude
what is the dimension formula of energy?
David Reply
what is viscosity?
David
what is inorganic
emma Reply
what is chemistry
Youesf Reply
what is inorganic
emma
Chemistry is a branch of science that deals with the study of matter,it composition,it structure and the changes it undergoes
Adjei
please, I'm a physics student and I need help in physics
Adjanou
chemistry could also be understood like the sexual attraction/repulsion of the male and female elements. the reaction varies depending on the energy differences of each given gender. + masculine -female.
Pedro
A ball is thrown straight up.it passes a 2.0m high window 7.50 m off the ground on it path up and takes 1.30 s to go past the window.what was the ball initial velocity
Krampah Reply
2. A sled plus passenger with total mass 50 kg is pulled 20 m across the snow (0.20) at constant velocity by a force directed 25° above the horizontal. Calculate (a) the work of the applied force, (b) the work of friction, and (c) the total work.
Sahid Reply
you have been hired as an espert witness in a court case involving an automobile accident. the accident involved car A of mass 1500kg which crashed into stationary car B of mass 1100kg. the driver of car A applied his brakes 15 m before he skidded and crashed into car B. after the collision, car A s
Samuel Reply
can someone explain to me, an ignorant high school student, why the trend of the graph doesn't follow the fact that the higher frequency a sound wave is, the more power it is, hence, making me think the phons output would follow this general trend?
Joseph Reply
Nevermind i just realied that the graph is the phons output for a person with normal hearing and not just the phons output of the sound waves power, I should read the entire thing next time
Joseph
Follow up question, does anyone know where I can find a graph that accuretly depicts the actual relative "power" output of sound over its frequency instead of just humans hearing
Joseph
"Generation of electrical energy from sound energy | IEEE Conference Publication | IEEE Xplore" ***ieeexplore.ieee.org/document/7150687?reload=true
Ryan
what's motion
Maurice Reply
what are the types of wave
Maurice
answer
Magreth
progressive wave
Magreth
hello friend how are you
Muhammad Reply
fine, how about you?
Mohammed
hi
Mujahid
A string is 3.00 m long with a mass of 5.00 g. The string is held taut with a tension of 500.00 N applied to the string. A pulse is sent down the string. How long does it take the pulse to travel the 3.00 m of the string?
yasuo Reply
Who can show me the full solution in this problem?
Reofrir Reply
Got questions? Join the online conversation and get instant answers!
Jobilize.com Reply
<< Chapter < Page Page > Chapter >>
Practice Key Terms 4

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

Get it on Google Play Download on the App Store Now




Source:  OpenStax, Physics subject knowledge enhancement course (ske). OpenStax CNX. Jan 09, 2015 Download for free at http://legacy.cnx.org/content/col11505/1.10
Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Physics subject knowledge enhancement course (ske)' conversation and receive update notifications?

Ask