1. Home
  2. Introductory physics - for kpu
  3. Uniform circular motion and
  4. Centripetal force

6.2 Centripetal force  (Page 5/10)

<< Chapter < Page
  Introductory physics - for kpu     Page 5 / 10
Chapter >> Page >

What is the ideal speed to take a 100 m radius curve banked at a 20.0° angle?

(a) What is the radius of a bobsled turn banked at 75.0° and taken at 30.0 m/s, assuming it is ideally banked?

(b) Calculate the centripetal acceleration.

(c) Does this acceleration seem large to you?

a) 24.6 m

b) 36.6 m / s 2 size 12{"36" "." 6m/s rSup { size 8{2} } } {}

c) a c = 3.73 g. This does not seem too large, but it is clear that bobsledders feel a lot of force on them going through sharply banked turns.

Part of riding a bicycle involves leaning at the correct angle when making a turn, as seen in [link] . To be stable, the force exerted by the ground must be on a line going through the center of gravity. The force on the bicycle wheel can be resolved into two perpendicular components—friction parallel to the road (this must supply the centripetal force), and the vertical normal force (which must equal the system’s weight).

(a) Show that θ size 12{θ} {} (as defined in the figure) is related to the speed v size 12{v} {} and radius of curvature r size 12{r} {} of the turn in the same way as for an ideally banked roadway—that is, θ = tan –1 v 2 / rg

(b) Calculate θ size 12{θ} {} for a 12.0 m/s turn of radius 30.0 m (as in a race).

The given figure shows a boy riding a bicycle, from the front. The boy is sliding leftward to his left. Three vectors are shown. One is from the bottom the front cycle wheel to the right depicting centripetal force, another one is from the same point drawn vertically upward showing the force N, making an angle theta with the slope of the front cycle wheel. The third vector is drawn from the chest of the boy to vertically downward to the bottom showing his weight, w. An arrow from the bottom of the wheel to the chest point of the boy is also shown depicting the slope of the bicycle with force F exerting on it. A free-body diagram is also given alongside the figure showing the direction of weight and force vectors. And the values of net F equals to sum of N and centripetal force, and N equals to weight W also given alongside on the right.
A bicyclist negotiating a turn on level ground must lean at the correct angle—the ability to do this becomes instinctive. The force of the ground on the wheel needs to be on a line through the center of gravity. The net external force on the system is the centripetal force. The vertical component of the force on the wheel cancels the weight of the system while its horizontal component must supply the centripetal force. This process produces a relationship among the angle θ , the speed v , and the radius of curvature r of the turn similar to that for the ideal banking of roadways.

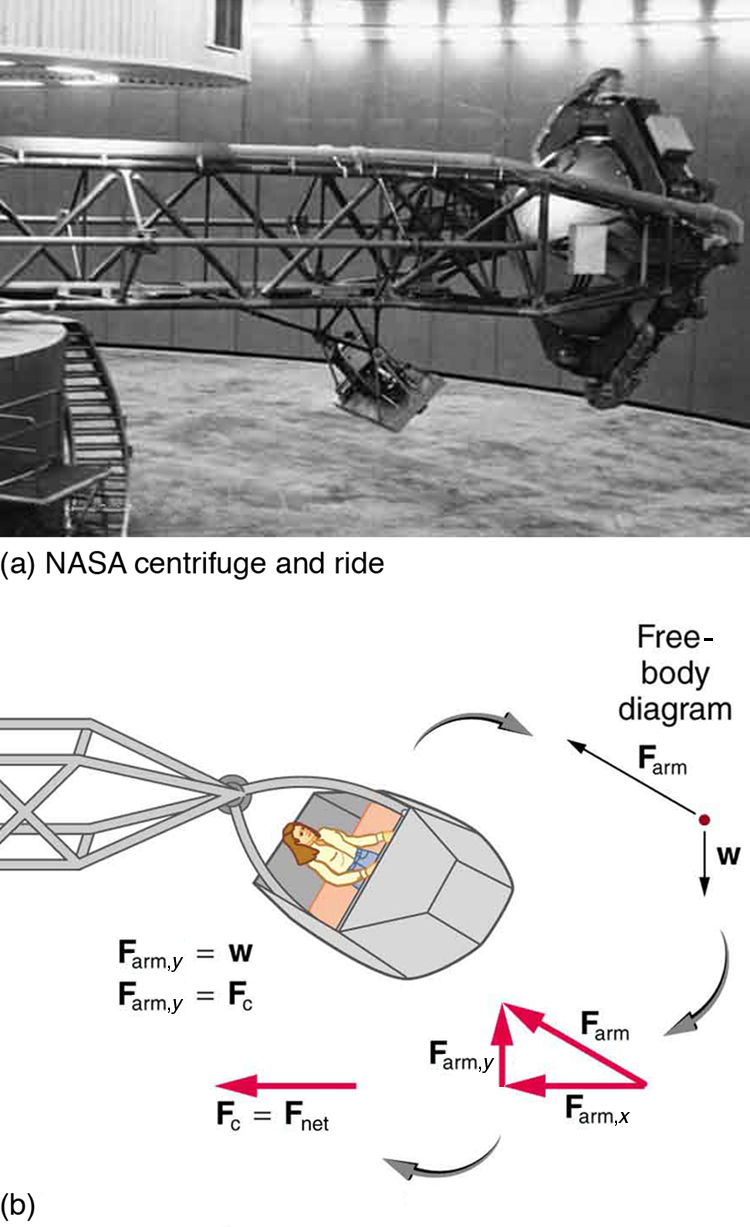
A large centrifuge, like the one shown in [link] (a), is used to expose aspiring astronauts to accelerations similar to those experienced in rocket launches and atmospheric reentries.

(a) At what angular velocity is the centripetal acceleration 10 g if the rider is 15.0 m from the center of rotation?

(b) The rider’s cage hangs on a pivot at the end of the arm, allowing it to swing outward during rotation as shown in [link] (b). At what angle θ size 12{θ} {} below the horizontal will the cage hang when the centripetal acceleration is 10 g ? (Hint: The arm supplies centripetal force and supports the weight of the cage. Draw a free body diagram of the forces to see what the angle θ size 12{θ} {} should be.)

Figure a shows a NASA centrifuge n a big hall. In figure b, there is a girl sitting in the cage of the centrifuge. The centripetal force on the cage is directed toward left. The direction of the weight of the cage is downward and the force on the arm is directed in north-west direction.
(a) NASA centrifuge used to subject trainees to accelerations similar to those experienced in rocket launches and reentries. (credit: NASA) (b) Rider in cage showing how the cage pivots outward during rotation. This allows the total force exerted on the rider by the cage to be along its axis at all times.

a) 2.56 rad/s

b) 5.71º size 12{5 cdot "71" rSup { size 8{0} } } {}

Integrated Concepts

If a car takes a banked curve at less than the ideal speed, friction is needed to keep it from sliding toward the inside of the curve (a real problem on icy mountain roads). (a) Calculate the ideal speed to take a 100 m radius curve banked at 15.0º. (b) What is the minimum coefficient of friction needed for a frightened driver to take the same curve at 20.0 km/h?

a) 16.2 m/s

b) 0.234

Modern roller coasters have vertical loops like the one shown in [link] . The radius of curvature is smaller at the top than on the sides so that the downward centripetal acceleration at the top will be greater than the acceleration due to gravity, keeping the passengers pressed firmly into their seats. What is the speed of the roller coaster at the top of the loop if the radius of curvature there is 15.0 m and the downward acceleration of the car is 1.50 g?

A teardrop shaped loop of a roller coaster is shown. The car of the roller coaster starts from the point A near the right of the base and covers the teardrop portion of the roller coaster and move to a point D at the left of base. Near the top of tear drop portion an upward arrow is shown labeled as r-minimum. Also at a point near the base toward A there is a label called r-maximum. The wire frame of the base is also shown.
Teardrop-shaped loops are used in the latest roller coasters so that the radius of curvature gradually decreases to a minimum at the top. This means that the centripetal acceleration builds from zero to a maximum at the top and gradually decreases again. A circular loop would cause a jolting change in acceleration at entry, a disadvantage discovered long ago in railroad curve design. With a small radius of curvature at the top, the centripetal acceleration can more easily be kept greater than g so that the passengers do not lose contact with their seats nor do they need seat belts to keep them in place.

Unreasonable Results

(a) Calculate the minimum coefficient of friction needed for a car to negotiate an unbanked 50.0 m radius curve at 30.0 m/s.

(b) What is unreasonable about the result?

(c) Which premises are unreasonable or inconsistent?

a) 1.84

b) A coefficient of friction this much greater than 1 is unreasonable .

c) The assumed speed is too great for the tight curve.
<< Chapter < Page Page > Chapter >>
Practice Key Terms 5

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

Get it on Google Play Download on the App Store Now




Source:  OpenStax, Introductory physics - for kpu phys 1100 (2015 edition). OpenStax CNX. May 30, 2015 Download for free at http://legacy.cnx.org/content/col11588/1.13
Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Introductory physics - for kpu phys 1100 (2015 edition)' conversation and receive update notifications?

Ask