5.6 Continuous random variables: continuous probability functions

Consider the function $f(x)=\frac{1}{20}$ for $0\le x\le \mathrm{20}$ . $x$ = a real number. The graph of $f(x)=\frac{1}{20}$ is a horizontal line. However, since $0\le x\le \mathrm{20}$ , $f(x)$ is restricted to the portion between $x=0$ and $x=20$ , inclusive .

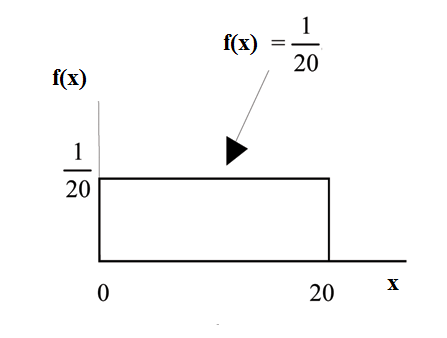
$f(x)=\frac{1}{20}$ for $0\le x\le 20$ .

The graph of $f(x)=\frac{1}{20}$ isa horizontal line segment when $0\le x\le \mathrm{20}$ .

The area between $f(x)=\frac{1}{20}$ where $0\le x\le \mathrm{20}$ and the x-axis is the area of a rectangle with base = $20$ and height = $\frac{1}{20}$ .

$\text{AREA}=20\cdot \frac{1}{20}=1$

This particular function, where we have restricted $x$ so that the area between the function and the x-axis is 1, is an example of a continuousprobability density function. It is used as a tool to calculate probabilities.

Suppose we want to find the area between $f(x)=\frac{1}{20}$ and the x-axis where $0< x< 2$ .

$\text{AREA}=(2-0)\cdot \frac{1}{20}=0.1$

$(2-0)=2=\text{base of a rectangle}$

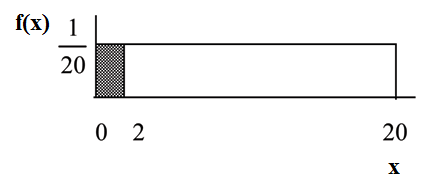
$\frac{1}{20}$ = the height.

The area corresponds to a probability. The probability that $x$ is between 0 and 2 is 0.1, which can be written mathematically as $\text{P(0<x<2)}=\text{P(x<2)}=0.1$ .

Suppose we want to find the area between $f(x)=\frac{1}{20}$ and the x-axis where $4< x< 15$ .

$\text{AREA}=(15-4)\cdot \frac{1}{20}=0.55$

$(15-4)=11=\text{the base of a rectangle}$

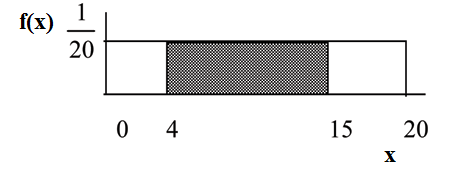
$\frac{1}{20}$ = the height.

The area corresponds to the probability $P(4< x< 15)=0.55$ .

Suppose we want to find $P(x=\mathrm{15})$ . On an x-y graph, $x=\mathrm{15}$ is a vertical line. A vertical line has no width (or 0 width). Therefore, $P(x=\mathrm{15})=\text{(base)}\text{(height)}=\left(0\right)\left(\frac{1}{20}\right)=0$ .

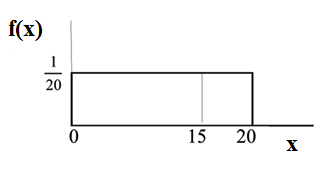
$P(X\le x)$ (can be written as $P(X< x)$ for continuous distributions) is called the cumulative distribution function or $\mathrm{CDF}$ . Notice the "less than or equal to" symbol. We can use the $\mathrm{CDF}$ to calculate $P(X> x)$ . The $\mathrm{CDF}$ gives "area to the left" and $P(X> x)$ gives "area to the right." We calculate $P(X> x)$ for continuous distributions as follows: $P(X> x)=1-P(X< x)$ .

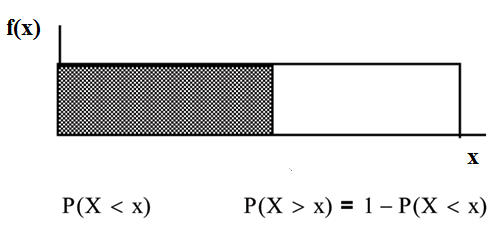
Label the graph with $\mathrm{f(x)}$ and $x$ . Scale the x and y axes with the maximum $x$ and $y$ values. $f(x)=\frac{1}{20}$ , $0\le x\le \mathrm{20}$ .

$P(2.3< x< 12.7)=\left(\text{base}\right)\left(\text{height}\right)=(12.7-2.3)\left(\frac{1}{20}\right)=0.52$