5.1 The straight line

Functions of the form $y=ax+q$

Functions with a general form of $y=ax+q$ are called straight line functions. In the equation, $y=ax+q$ , $a$ and $q$ are constants and have different effects on the graph of the function. The general shape of the graph of functions of this form is shown in [link] for the function $f\left(x\right)=2x+3$ .

Investigation : functions of the form $y=ax+q$

1. On the same set of axes, plot the following graphs:
   1. $a\left(x\right)=x-2$
   2. $b\left(x\right)=x-1$
   3. $c\left(x\right)=x$
   4. $d\left(x\right)=x+1$
   5. $e\left(x\right)=x+2$
   Use your results to deduce the effect of different values of $q$ on the resulting graph.
2. On the same set of axes, plot the following graphs:
   1. $f\left(x\right)=-2·x$
   2. $g\left(x\right)=-1·x$
   3. $h\left(x\right)=0·x$
   4. $j\left(x\right)=1·x$
   5. $k\left(x\right)=2·x$
   Use your results to deduce the effect of different values of $a$ on the resulting graph.

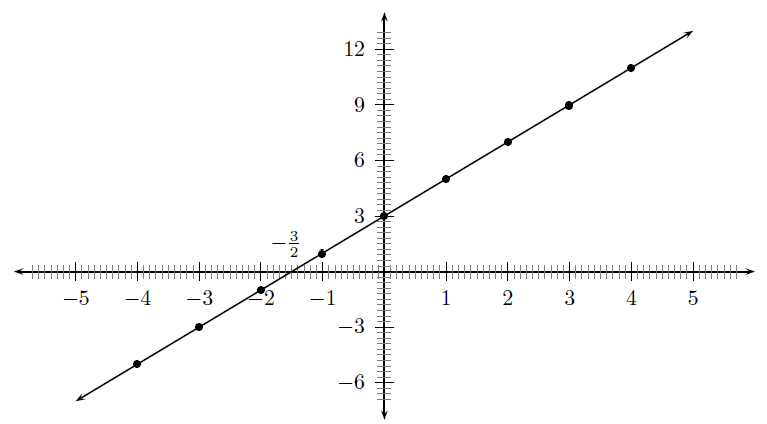
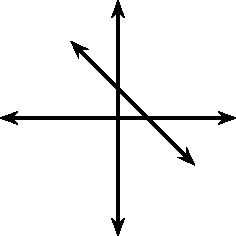
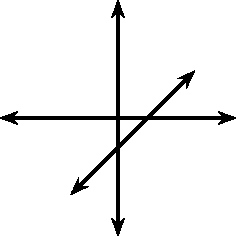
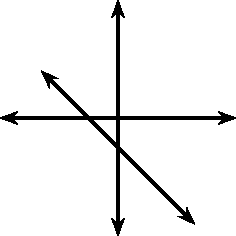
You may have noticed that the value of $a$ affects the slope of the graph. As $a$ increases, the slope of the graph increases. If $a>0$ then the graph increases from left to right (slopes upwards). If $a<0$ then the graph increases from right to left (slopes downwards). For this reason, $a$ is referred to as the slope or gradient of a straight-line function.

You should have also found that the value of $q$ affects where the graph passes through the $y$ -axis. For this reason, $q$ is known as the y-intercept .

These different properties are summarised in [link] .

	$a>0$	$a<0$
$q>0$
$q<0$

Domain and range

For $f\left(x\right)=ax+q$ , the domain is $\{x:x\in \mathbb{R}\}$ because there is no value of $x\in \mathbb{R}$ for which $f\left(x\right)$ is undefined.

The range of $f\left(x\right)=ax+q$ is also $\left\{f\right(x):f(x)\in \mathbb{R}\}$ because there is no value of $f\left(x\right)\in \mathbb{R}$ for which $f\left(x\right)$ is undefined.

For example, the domain of $g\left(x\right)=x-1$ is $\{x:x\in \mathbb{R}\}$ because there is no value of $x\in \mathbb{R}$ for which $g\left(x\right)$ is undefined. The range of $g\left(x\right)$ is $\left\{g\right(x):g(x)\in \mathbb{R}\}$ .

Intercepts

For functions of the form, $y=ax+q$ , the details of calculating the intercepts with the $x$ and $y$ axis are given.

The $y$ -intercept is calculated as follows:

For example, the $y$ -intercept of $g\left(x\right)=x-1$ is given by setting $x=0$ to get:

The $x$ -intercepts are calculated as follows:

For example, the $x$ -intercepts of $g\left(x\right)=x-1$ is given by setting $y=0$ to get:

Turning points

The graphs of straight line functions do not have any turning points.

Axes of symmetry

The graphs of straight-line functions do not, generally, have any axes of symmetry.

Sketching graphs of the form $f\left(x\right)=ax+q$

In order to sketch graphs of the form, $f\left(x\right)=ax+q$ , we need to determine three characteristics:

1. sign of $a$
2. $y$ -intercept
3. $x$ -intercept

Only two points are needed to plot a straight line graph. The easiest points to use are the $x$ -intercept (where the line cuts the $x$ -axis) and the $y$ -intercept.

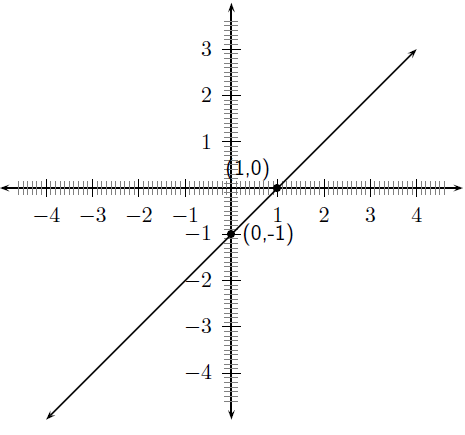
For example, sketch the graph of $g\left(x\right)=x-1$ . Mark the intercepts.

Firstly, we determine that $a>0$ . This means that the graph will have an upward slope.

The $y$ -intercept is obtained by setting $x=0$ and was calculated earlier to be $y_{int}=-1$ . The $x$ -intercept is obtained by setting $y=0$ and was calculated earlier to be $x_{int}=1$ .

Exercise: straight line graphs

1. List the $y$ -intercepts for the following straight-line graphs:
   1. $y=x$
   2. $y=x-1$
   3. $y=2x-1$
   4. $y+1=2x$
2. Give the equation of the illustrated graph below:

   

3. Sketch the following relations on the same set of axes, clearly indicating the intercepts with the axes as well as the co-ordinates of the point of interception of the graph: $x+2y-5=0$ and $3x-y-1=0$