4.7 Nonconservative forces  (Page 6/3)

Nonconservative forces and friction

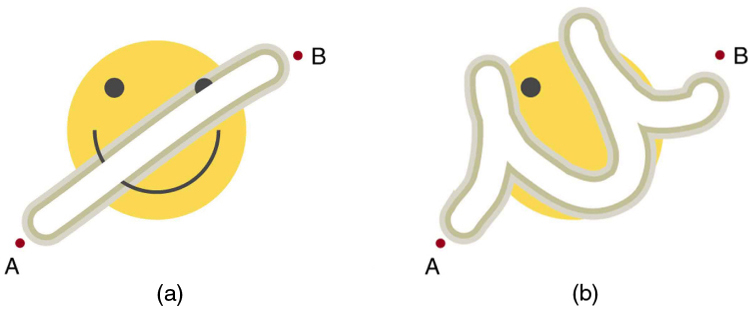
Forces are either conservative or nonconservative. Conservative forces were discussed in "Conservative Forces and Potential Energy". A nonconservative force    is one for which work depends on the path taken. Friction is a good example of a nonconservative force. As illustrated in [link] , work done against friction depends on the length of the path between the starting and ending points. Because of this dependence on path, there is no potential energy associated with nonconservative forces. An important characteristic is that the work done by a nonconservative force adds or removes mechanical energy from a system . Friction , for example, creates thermal energy    that dissipates, removing energy from the system. Furthermore, even if the thermal energy is retained or captured, it cannot be fully converted back to work, so it is lost or not recoverable in that sense as well.

How nonconservative forces affect mechanical energy

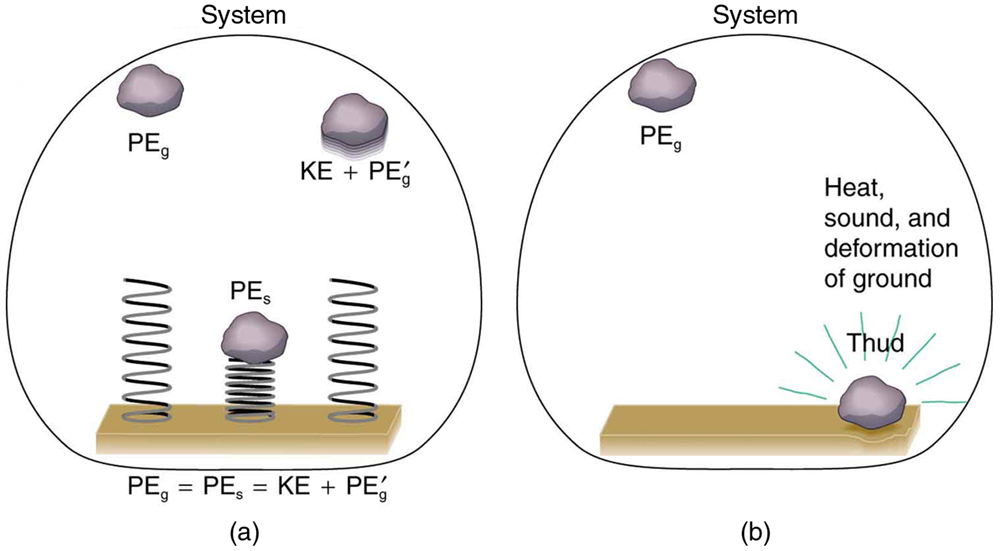
Mechanical energy may not be conserved when nonconservative forces act. For example, when a car is brought to a stop by friction on level ground, it loses kinetic energy, which is dissipated as thermal energy, reducing its mechanical energy. [link] compares the effects of conservative and nonconservative forces. We often choose to understand simpler systems such as that described in [link] (a) first before studying more complicated systems as in [link] (b).

How the work-energy theorem applies

Now let us consider what form the work-energy theorem takes when both conservative and nonconservative forces act. We will see that the work done by nonconservative forces equals the change in the mechanical energy of a system. As noted in "Kinetic Energy and the Work-Energy Theorem", the work-energy theorem states that the net work on a system equals the change in its kinetic energy, or $W_{\text{net}}=\text{ΔKE}$ . The net work is the sum of the work by nonconservative forces plus the work by conservative forces. That is,

so that

where $W_{\text{nc}}$ is the total work done by all nonconservative forces and $W_{\text{c}}$ is the total work done by all conservative forces.

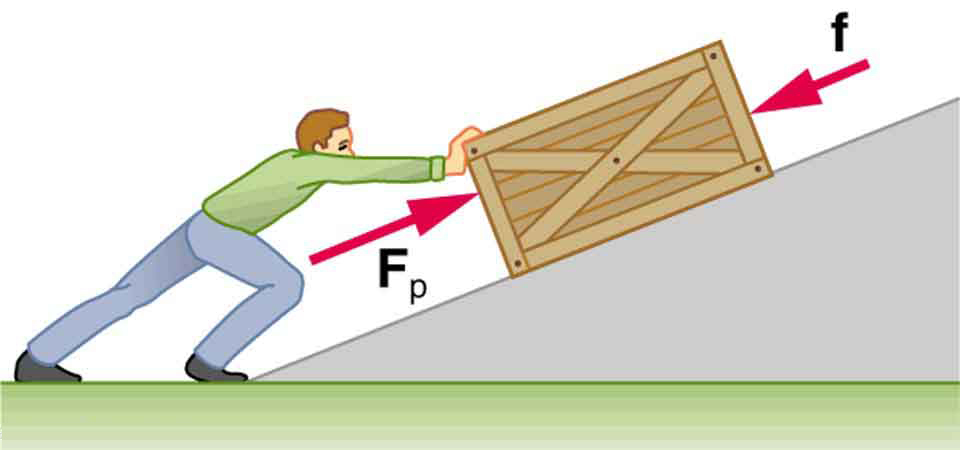
Consider [link] , in which a person pushes a crate up a ramp and is opposed by friction. As in the previous section, we note that work done by a conservative force comes from a loss of gravitational potential energy, so that $W_{\text{c}}=-\text{Δ}\text{PE}$ . Substituting this equation into the previous one and solving for $W_{\text{nc}}$ gives

This equation means that the total mechanical energy $(\text{KE + PE})$ changes by exactly the amount of work done by nonconservative forces. In [link] , this is the work done by the person minus the work done by friction. So even if energy is not conserved for the system of interest (such as the crate), we know that an equal amount of work was done to cause the change in total mechanical energy.

We rearrange $W_{\text{nc}}=\text{Δ}\text{KE}+\text{Δ}\text{PE}$ to obtain

This means that the amount of work done by nonconservative forces adds to the mechanical energy of a system. If $W_{\text{nc}}$ is positive, then mechanical energy is increased, such as when the person pushes the crate up the ramp in [link] . If $W_{\text{nc}}$ is negative, then mechanical energy is decreased, such as when the rock hits the ground in [link] (b). If $W_{\text{nc}}$ is zero, then mechanical energy is conserved, and nonconservative forces are balanced. For example, when you push a lawn mower at constant speed on level ground, your work done is removed by the work of friction, and the mower has a constant energy.

Section summary

• A nonconservative force is one for which work depends on the path.
• Friction is an example of a nonconservative force that changes mechanical energy into thermal energy.
• Work $W_{\text{nc}}$ done by a nonconservative force changes the mechanical energy of a system. In equation form, $W_{\text{nc}}=\text{Δ}\text{KE}+\text{Δ}\text{PE}$ or, equivalently, ${\text{KE}}_{\text{i}}+{\text{PE}}_{\text{i}}+W_{\text{nc}}={\text{KE}}_{\text{f}}+{\text{PE}}_{\text{f}}$ .
• When both conservative and nonconservative forces act, energy conservation can be applied and used to calculate motion in terms of the known potential energies of the conservative forces and the work done by nonconservative forces, instead of finding the net work from the net force, or having to directly apply Newton’s laws.