4.5 Geometric calculus of variations: vigre summer 2011

Introduction

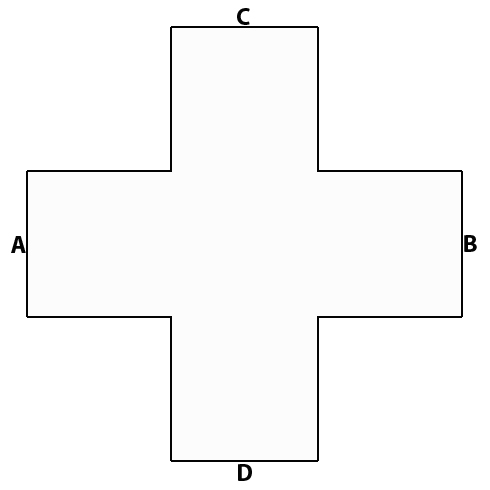
The primary objective of the PFUG was to study extremal metrics on the Swiss Cross, which is defined to be five unit squares arranged in a cross (Figure 1).

Let $\Gamma =\left\{\right(\text{Connected}\text{curves}\text{between}A\text{and}B)\cup (\text{Connected}\text{curves}\text{between}C\text{and}D\left)\right\}$ . Then we wish to find a metric $\rho (x,y)$ on the Swiss Cross (S.C.) which minimizes

subject to the constraint

Another problem of interest is finding the extremal metric on a unit disk where ${\Gamma }^{\text{'}}=\left\{\text{Connected}\text{curves}\text{between}\text{antipodal}\text{points}\text{on}\text{the}\text{boundary}\right\}$ . Again we are trying to minimize

on $\Delta =$ the unit disk, subject to the constraint that

Definitions

Definition 1 A functional is a mapping from a function space to the real numbers. We are particularly interested in the maps $F$ : $L^2\left(\Re \right)$ $\to$ $\Re$ .

Definition 2 The Area functional, $A_{\rho }\left(\Omega \right)$ , is defined to be:

where $\Omega$ is our region of interest.

Definition 3 The $Length$ of $\Gamma$ with respect to a metric $\rho$ is defined to be:

where the $\gamma$ are rectifiable curves in the set of curves $\Gamma$ .

Definition 4 The Extremal Length of a region $\Omega$ with respect to a set of curves $\Gamma$ contained within $\Omega$ is defined to be:

where $\rho \in L^2\left(\Re \right)$ and $\rho$ : ${\Re }^2\to {\Re }_{+}$

Previous results

There has not been very much work done on the topic of extremal length, and thus, most of the previous results are limited to one source by Ahlfors. Nevertheless there are still many useful theorems that we can apply to our topic. The following are two theorems from [1].

Theorem 1 Let us say that a metric $\rho (x,y)$ is admissible if $L_{\rho }\left(\Gamma \right)\ge 1$ . Then the extremal length is equal to $\frac{1}{inf{A}_{\rho }\left(\Omega \right)}$ .

This can easily be applied to our Swiss Cross case, as instead of defining an admissible metric as one where $L_{\rho }\left(\Gamma \right)\ge 1$ , we can define it as $L_{\rho }\left(\Gamma \right)\ge 3$ .

Another result is one that lets us actually check if a metric $\rho (x,y)$ is an extremal metric.

Theorem 2 The metric ${\rho }_0$ is extremal for $\Gamma$ on a region $\Omega$ if $\Gamma$ contains a subfamily ${\Gamma }_0$ with the following properties:

And secondly, for any real-valued $h$ on $\Omega$ , $h$ satisfying

for all $\gamma \in {\Gamma }_0$ implies:

This is useful because it states that the set of extremal curves, ${\Gamma }_0$ covers the whole region $\Omega$ except possibly where ${\rho }_0$ is equal to zero.

Results

Swiss cross

Preliminary inspection of extremal metrics yields a constant value of $\rho (x,y)=1$ as a function which satisfies the constraints and has metric area 5. However, $\rho \equiv 1$ is not extremal for the Swiss Cross. This can be shown by the function in Figure 2.

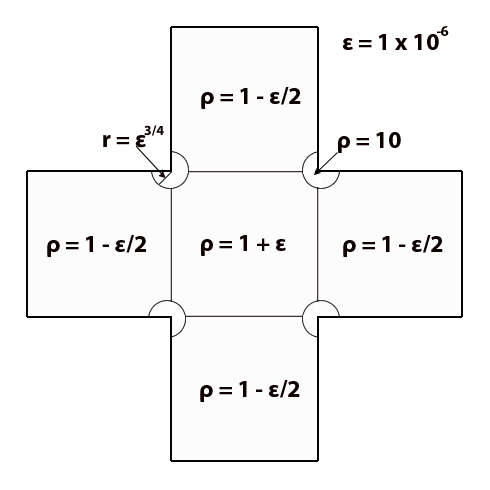
Theorem 3 The function in Figure 2 satisfies the constraints of the extremal length problem, and has a smaller metric area than $\rho \equiv 1$ .