| << Chapter < Page | Chapter >> Page > |
A cleaner pushes a 4.50-kg laundry cart in such a way that the net external force on it is 60.0 N. Calculate the magnitude of its acceleration.
Since astronauts in orbit are apparently weightless, a clever method of measuring their masses is needed to monitor their mass gains or losses to adjust diets. One way to do this is to exert a known force on an astronaut and measure the acceleration produced. Suppose a net external force of 50.0 N is exerted and the astronaut’s acceleration is measured to be . (a) Calculate her mass. (b) By exerting a force on the astronaut, the vehicle in which they orbit experiences an equal and opposite force. Discuss how this would affect the measurement of the astronaut’s acceleration. Propose a method in which recoil of the vehicle is avoided.
In [link] , the net external force on the 24-kg mower is stated to be 51 N. If the force of friction opposing the motion is 24 N, what force (in newtons) is the person exerting on the mower? Suppose the mower is moving at 1.5 m/s when the force is removed. How far will the mower go before stopping?
The same rocket sled drawn in [link] is decelerated at a rate of . What force is necessary to produce this deceleration? Assume that the rockets are off. The mass of the system is 2100 kg.

(a) If the rocket sled shown in [link] starts with only one rocket burning, what is the magnitude of its acceleration? Assume that the mass of the system is 2100 kg, the thrust T is N, and the force of friction opposing the motion is known to be 650 N. (b) Why is the acceleration not one-fourth of what it is with all rockets burning?
(a) .
(b) The acceleration is not one-fourth of what it was with all rockets burning because the frictional force is still as large as it was with all rockets burning.
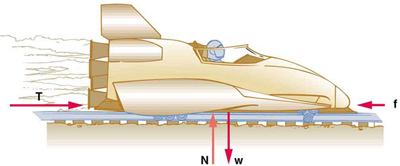
What is the deceleration of the rocket sled if it comes to rest in 1.1 s from a speed of 1000 km/h? (Such deceleration caused one test subject to black out and have temporary blindness.)
Suppose two children push horizontally, but in exactly opposite directions, on a third child in a wagon. The first child exerts a force of 75.0 N, the second a force of 90.0 N, friction is 12.0 N, and the mass of the third child plus wagon is 23.0 kg. (a) What is the system of interest if the acceleration of the child in the wagon is to be calculated? (b) Draw a free-body diagram, including all forces acting on the system. (c) Calculate the acceleration. (d) What would the acceleration be if friction were 15.0 N?
(a) The system is the child in the wagon plus the wagon.
(b
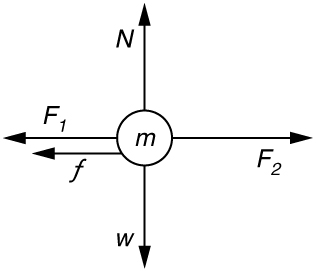
(c) in the direction of the second child’s push.
(d)
A powerful motorcycle can produce an acceleration of while traveling at 90.0 km/h. At that speed the forces resisting motion, including friction and air resistance, total 400 N. (Air resistance is analogous to air friction. It always opposes the motion of an object.) What is the magnitude of the force the motorcycle exerts backward on the ground to produce its acceleration if the mass of the motorcycle with rider is 245 kg?
The rocket sled shown in [link] accelerates at a rate of . Its passenger has a mass of 75.0 kg. (a) Calculate the horizontal component of the force the seat exerts against his body. Compare this with his weight by using a ratio. (b) Calculate the direction and magnitude of the total force the seat exerts against his body.
(a) . This force is 5.00 times greater than his weight.
(b)

Repeat the previous problem for the situation in which the rocket sled decelerates at a rate of . In this problem, the forces are exerted by the seat and restraining belts.
The weight of an astronaut plus his space suit on the Moon is only 250 N. How much do they weigh on Earth? What is the mass on the Moon? On Earth?
Suppose the mass of a fully loaded module in which astronauts take off from the Moon is 10,000 kg. The thrust of its engines is 30,000 N. (a) Calculate its the magnitude of acceleration in a vertical takeoff from the Moon. (b) Could it lift off from Earth? If not, why not? If it could, calculate the magnitude of its acceleration.

Notification Switch
Would you like to follow the 'Abe advanced level physics' conversation and receive update notifications?