2.9 Circular motion with constant acceleration (application)  (Page 2/2)

Problem : The angular position of a point on a flywheel is given by the relation :

$$\begin{array}{l}\theta \mathrm{(rad)}=-0.025t^2+0.01t\end{array}$$

Find the time (in seconds) when flywheel comes to a stop.

Solution : The speed of the particle is :

$$\begin{array}{l}\omega =\frac{d\theta }{dt}=-0.025X2t+0.1\end{array}$$

When flywheel comes to a standstill, ω = 0,

$$\begin{array}{l}\Rightarrow 0=-0.025X2t+0.1\end{array}$$

$$\begin{array}{l}\Rightarrow t=\frac{0.1}{0.025}=\frac{100}{25}=4s\end{array}$$

Problem : The magnitude of deceleration of the motion of a point on a rotating disk is equal to the acceleration due to gravity (10 $m/s^2$ ). The point is at a linear distance 10 m from the center of the disk. If initial speed is 40 m/s in anti-clockwise direction, then find the time for the point to return to its position.

Solution : The disk first rotates in anti-clockwise direction till its speed becomes zero and then the disk turns back to move in clockwise direction. In order to analyze the motion, we first convert linear quantities to angular quantities as :

$${\omega }_i=\frac{v_i}{r}=\frac{40}{10}=4\mathrm{rad}/s$$

$$\alpha =\frac{a_T}{r}=\frac{10}{10}=1\mathrm{rad}/s^2$$

When the point returns to its initial position, the total displacement is zero. Applying equation of motion for angular displacement, we have :

$$\theta ={\omega }_{i}t+\frac{1}{2}\alpha t^2$$

$$\Rightarrow 0=4Xt-\frac{1}{2}X1X{t}^2$$

$$\Rightarrow t^2-8t=0$$

$$\Rightarrow t\left(t-8\right)=0$$

$$\Rightarrow t=0or8s$$

The zero time corresponds to initial position. The time of return to initial position, therefore, is 8 seconds.

Problem : A disk initially rotating at 80 rad/s is slowed down with a constant deceleration of magnitude 4 $\mathrm{rad}/s^2$ . What angle (rad) does the disk rotate before coming to rest ?

Solution : Initial and final angular velocities and angular acceleration are given. We can use $\omega ={\omega }_0+\alpha t$ to determine the time disk takes to come to stop. Here,

$$\begin{array}{l}{\omega }_0=80\mathrm{rad}/s,\alpha =4\mathrm{rad}/s^2\end{array}$$

$$\begin{array}{l}\Rightarrow 0=80-4t\\ \Rightarrow t=20s\end{array}$$

Using equation, $\theta ={\omega }_{0}t+\frac{1}{2}\alpha t^2$ , we have :

$$\begin{array}{l}\Rightarrow \theta =80X20-\frac{1}{2}X4X{20}^2=1600-800=800rad\end{array}$$

Problem : The initial angular velocity of a point (in radian) on a rotating disk is 0.5 rad/s. The disk is subjected to a constant acceleration of 0. 2 $\mathrm{rad}/s^2$ . in the direction opposite to the angular velocity. Determine the angle (in radian) through which the point moves in third second.

Solution : Here, we need to be careful as the point reverses its direction in the third second ! For ω = 0,

$$\begin{array}{l}\omega ={\omega }_0+\alpha t\\ \Rightarrow 0=0.5-0.2t\\ \Rightarrow t=\frac{0.5}{0.2}=2.5s\end{array}$$

Thus the disk stops at t = 2.5 second. In this question, we are to find the angle (in rad) through which the point moves in third second – not the displacement. The figure here qualitatively depicts the situation. In the third second, the point moves from B to C and then from C to D. The displacement in third second is BOD, whereas the angle moved in the third second is |BOC| + |DOC|. Where,

∠BOC = displacement between 2 and 2.5 seconds.

∠DOC = displacement between 2.5 and 3 seconds.

Angle measurement

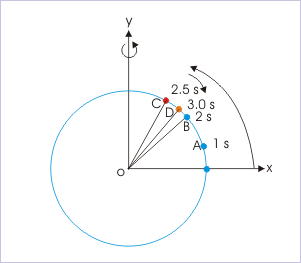
The angular velocity at the end of 2 seconds is :

$$\begin{array}{l}\omega =0.5-0.2x2=0.1\mathrm{rad}/s\end{array}$$

$$\begin{array}{l}\angle \mathrm{BOC}=\omega t-\frac{1}{2}\alpha t^2\\ \Rightarrow \angle \mathrm{BOC}=0.1X0.5-\frac{1}{2}X0.2X{0.5}^2\\ \Rightarrow \angle \mathrm{BOC}=0.05-0.1X0.25=0.05-0.025=0.025\mathrm{rad}\end{array}$$

The angular velocity at the end of 2.5 seconds is zero. Hence,

$$\begin{array}{l}\Rightarrow \angle \mathrm{DOC}=0-\frac{1}{2}x0.2x{0.5}^2\\ \Rightarrow \angle \mathrm{BOC}=-0.1x0.25=-0.025\mathrm{rad}\end{array}$$

It means that the point, at t = 3 s, actually returns to the position where it was at t = 2 s. The displacement is, thus, zero.

The total angle moved in third second = |0.025| + |-0.025| = 0.05 rad

Problem : The angular velocity of a point (in radian) on a rotating disk is given by |t – 2|, where “t” is in seconds. If the point aligns with the reference direction at time t = 0, then find the quadrant in which the point falls after 5 seconds.

Problem : The area under the angular velocity – time plot and time axis is equal to angular displacement. As required, let us generate angular velocity data for first 5 seconds to enable us draw the requisite plot :

--------------------------------- Time (t) Angular velocity (θ)(s) (rad/s) ---------------------------------0 2 1 12 0 3 14 2 5 3---------------------------------

The angular velocity – time plot is as shown in the figure :

Angular velocity – time plot

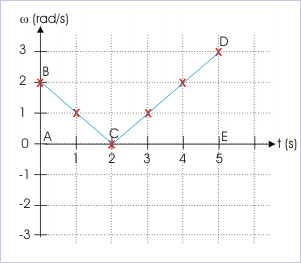
The displacement is equal to the area of two triangles :

$$\begin{array}{l}\Rightarrow \theta =\frac{1}{2}X2X2+\frac{1}{2}X3X3=6.5\mathrm{rad}\end{array}$$

Thus, the point moves 6.5 rad from the reference direction. Now, one revolution is equal to 2π = 2 x 3.14 = 6.28 rad. The particle is, therefore, in the fourth quadrant with respect to the reference direction.