| << Chapter < Page | Chapter >> Page > |
The equation reflects the fact that, when acceleration is constant, is just the simple average of the initial and final velocities. For example, if you steadily increase your velocity (that is, with constant acceleration) from 30 to 60 km/h, then your average velocity during this steady increase is 45 km/h. Using the equation to check this, we see that
which seems logical.
A jogger runs down a straight stretch of road with an average velocity of 4.00 m/s for 2.00 min. What is his final position, taking his initial position to be zero?
Strategy
Draw a sketch.

The final position is given by the equation
To find , we identify the values of , , and from the statement of the problem and substitute them into the equation.
Solution
1. Identify the knowns. , , and .
2. Enter the known values into the equation.
Discussion
Velocity and final displacement are both positive, which means they are in the same direction.
The equation gives insight into the relationship between displacement, average velocity, and time. It shows, for example, that displacement is a linear function of average velocity. (By linear function, we mean that displacement depends on rather than on raised to some other power, such as . When graphed, linear functions look like straight lines with a constant slope.) On a car trip, for example, we will get twice as far in a given time if we average 90 km/h than if we average 45 km/h.
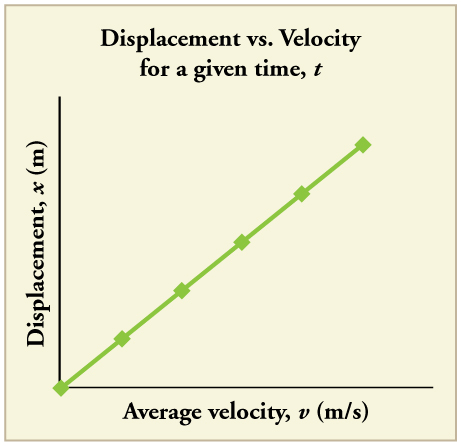
We can derive another useful equation by manipulating the definition of acceleration.
Substituting the simplified notation for and gives us
Solving for yields
An airplane lands with an initial velocity of 70.0 m/s and then decelerates at for 40.0 s. What is its final velocity?
Strategy
Draw a sketch. We draw the acceleration vector in the direction opposite the velocity vector because the plane is decelerating.
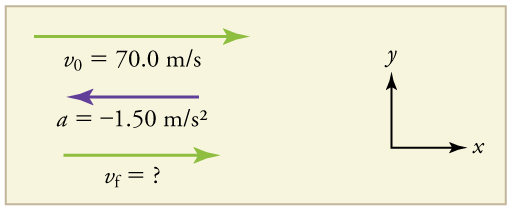
Solution
1. Identify the knowns. , , .
2. Identify the unknown. In this case, it is final velocity, .
3. Determine which equation to use. We can calculate the final velocity using the equation .
4. Plug in the known values and solve.
Discussion
The final velocity is much less than the initial velocity, as desired when slowing down, but still positive. With jet engines, reverse thrust could be maintained long enough to stop the plane and start moving it backward. That would be indicated by a negative final velocity, which is not the case here.


Notification Switch
Would you like to follow the 'Physics subject knowledge enhancement course (ske)' conversation and receive update notifications?