| << Chapter < Page | Chapter >> Page > |
4.2 How would you calculate the diameter of a circle when the circumference is provided?
Now you should be able to answer any question dealing with the diameter, radius or circumference of a circle or wheel or any circular object.
5. Use your pocket calculator to calculate the circumference of each of the following circles:
Note this : Always write out the formula before you start.(π = 3,14).
5.1 r = 230 mm
5.2 r = 1,45 cm (answer to 2 decimal figures)
6. Determine the circumference of each of the following without the use of a pocket calculator.
Note this : Always write out the formula before you start.(π = )
6.1 r = 14 cm
6.2 d = 35 cm
You may use your pocket calculator, but you have to show all the steps of the calculation. (π= )
7.1 circumference 242 mm
8. How many rotations will the wheel of a mountain bike complete over a distance of 7,5 m if the diameter of the wheel is 67 cm?
1. Can you remember the formula for calculating the area of a rectangle?
2. Draw a circle with centre O and a radius of 60 mm on a sheet of paper. Divide the circle into 32 equal sectors. Use red for colouring 16 sectors and blue for the remaining 16 sectors.
3. Cut out all 32 sectors and arrange them in line in such a way that the segments eventually form a rectangular paving design.
Paste your triangles in the following space
4. Measure both the length and breadth of the rectangle. Use the formula from no. 1 to calculate the area of the rectangle.
5. What do you deduce with regard to the rectangle and the circle that you have drawn in no. 2?
6. Which unit of measurement is used for calculating area?
7. Provide the formula for calculating the area of any circle.
8. Calculate the area of the circle you have drawn in no. 2 with the help of the formula from no. 7.
What do you notice?
9. Calculate the area of each of the following circles without making use of a pocket calculator.
9.1 r = 14,7 cm 9.2 d = 56,49 cm
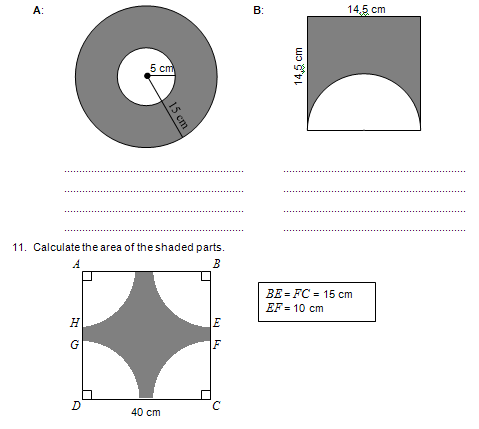
10. Calculate the area of the shaded parts.
| LO4 |
| MeasurementThe learner will be able to use appropriate measuring units, instruments and formulae in a variety of contexts. |
| We know this when the learner: |
| 4.2 solves problems involving: |
| 4.2.1 length; |
| 4.2.2 perimeter and area of polygonals and circles; |
| 4.3 solves problems using a range of strategies including: |
| 4.3.1 estimating; |
| 4.3.2 calculating to at least two decimal positions; |
| 4.3.3 using and converting between appropriate SI units; |
| 4.4 describes the meaning of and uses in calculations involving circles and discusses its historical development in measurement; |
| 4.5 calculates, by selecting and using appropriate formulae: |
| 4.5.1 perimeter of polygons and circles; |
| 4.5.2 area of triangles, rectangles circles and polygons by decomposition into triangles and rectangles; |
|
| 4.9 uses the Theorem of Pythagoras to calculate a missing length in a right-angled triangle leaving irrational answers in surd form (√); |
| 4.10 describes and illustrates ways of measuring in different cultures throughout history (e.g. determining right angles using knotted string leading to the Theorem of Pythagoras). |
ACTIVITY 2
5.1 O = x d
O = x 460
O = 1 444,4 mm
5.2 C = x d
C = x 2,9
C 9,11 cm
6.1 C = x d
C = x
C = 88 cm
6.2 C = x d
C = x
C = 110 cm
7.1 C = x d
242 = x d
x = d
d = 77 mm
8. C = x d 750 ÷ 210,38 cm
= 3,14 x 67 cm = 3,6 revolutions
= 210,38 cm
ACTIVITY 3
9. A = x r 2
= x x
= 679,14 cm 2
A = 2 505,92 cm 2
10. A B
(3,14 x 15 2 ) – (3,14 x 15 2 ) (14,5) 2 – (3,14 x 7,25 2 x )
= 706,5 – 78,5 = 210,25 – 82,52
= 628 cm 2 = 127,73 cm 2
11. (40 x 40) – (3,14 x 15 2 )
= 1 600 – 706,5
= 893,5 cm 2

Notification Switch
Would you like to follow the 'Mathematics grade 8' conversation and receive update notifications?