1. Home
  2. Physics 101
  3. Electric current, resistance,
  4. Resistors in series and parallel

14.6 Resistors in series and parallel  (Page 8/17)

<< Chapter < Page
  Physics 101     Page 8 / 17
Chapter >> Page >

If two household lightbulbs rated 60 W and 100 W are connected in series to household power, which will be brighter? Explain.

Suppose you are doing a physics lab that asks you to put a resistor into a circuit, but all the resistors supplied have a larger resistance than the requested value. How would you connect the available resistances to attempt to get the smaller value asked for?

Before World War II, some radios got power through a “resistance cord” that had a significant resistance. Such a resistance cord reduces the voltage to a desired level for the radio’s tubes and the like, and it saves the expense of a transformer. Explain why resistance cords become warm and waste energy when the radio is on.

Some light bulbs have three power settings (not including zero), obtained from multiple filaments that are individually switched and wired in parallel. What is the minimum number of filaments needed for three power settings?

Problem exercises

Note: Data taken from figures can be assumed to be accurate to three significant digits.

(a) What is the resistance of ten 275-Ω size 12{"275"- %OMEGA } {} resistors connected in series? (b) In parallel?

(a) 2 . 75 k Ω size 12{2 "." "75"" k" %OMEGA } {}

(b) 27 . 5 Ω size 12{"27" "." 5 %OMEGA } {}

(a) What is the resistance of a 1.00 × 10 2 -Ω , a 2 . 50-kΩ , and a 4 . 00-k Ω size 12{4 "." "00""-k" %OMEGA } {} resistor connected in series? (b) In parallel?

What are the largest and smallest resistances you can obtain by connecting a 36 . 0-Ω , a 50 . 0-Ω size 12{"50" "." 0- %OMEGA } {} , and a 700-Ω size 12{"700"- %OMEGA } {} resistor together?

(a) 786 Ω size 12{"786" %OMEGA } {}

(b) 20 . 3 Ω size 12{"20" "." 3 %OMEGA } {}

An 1800-W toaster, a 1400-W electric frying pan, and a 75-W lamp are plugged into the same outlet in a 15-A, 120-V circuit. (The three devices are in parallel when plugged into the same socket.). (a) What current is drawn by each device? (b) Will this combination blow the 15-A fuse?

Your car’s 30.0-W headlight and 2.40-kW starter are ordinarily connected in parallel in a 12.0-V system. What power would one headlight and the starter consume if connected in series to a 12.0-V battery? (Neglect any other resistance in the circuit and any change in resistance in the two devices.)

29 . 6 W size 12{"29" "." 6" W"} {}

(a) Given a 48.0-V battery and 24 . 0-Ω size 12{"24" "." 0- %OMEGA } {} and 96 . 0-Ω size 12{"96" "." 0- %OMEGA } {} resistors, find the current and power for each when connected in series. (b) Repeat when the resistances are in parallel.

Referring to the example combining series and parallel circuits and [link] , calculate I 3 size 12{I rSub { size 8{3} } } {} in the following two different ways: (a) from the known values of I size 12{I} {} and I 2 size 12{I rSub { size 8{2} } } {} ; (b) using Ohm’s law for R 3 size 12{R rSub { size 8{3} } } {} . In both parts explicitly show how you follow the steps in the Problem-Solving Strategies for Series and Parallel Resistors .

(a) 0.74 A

(b) 0.742 A

Referring to [link] : (a) Calculate P 3 size 12{P rSub { size 8{3} } } {} and note how it compares with P 3 size 12{P rSub { size 8{3} } } {} found in the first two example problems in this module. (b) Find the total power supplied by the source and compare it with the sum of the powers dissipated by the resistors.

Refer to [link] and the discussion of lights dimming when a heavy appliance comes on. (a) Given the voltage source is 120 V, the wire resistance is 0 . 400 Ω size 12{0 "." "800" %OMEGA } {} , and the bulb is nominally 75.0 W, what power will the bulb dissipate if a total of 15.0 A passes through the wires when the motor comes on? Assume negligible change in bulb resistance. (b) What power is consumed by the motor?

(a) 60.8 W

(b) 3.18 kW

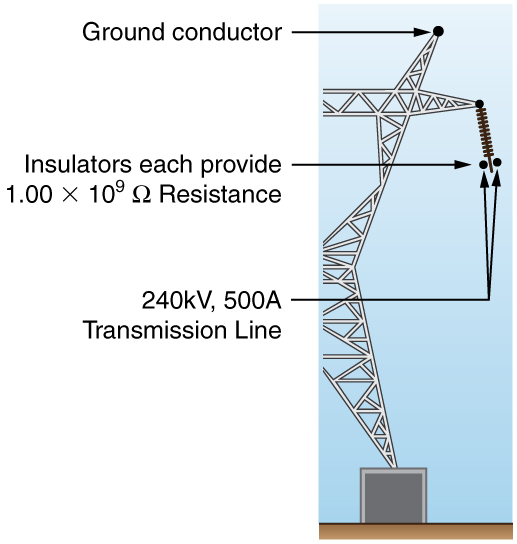
A 240-kV power transmission line carrying 5.00 × 10 2 A is hung from grounded metal towers by ceramic insulators, each having a 1 . 00 × 10 9 -Ω size 12{1 "." "00"´"10" rSup { size 8{9} } - %OMEGA } {} resistance. [link] . (a) What is the resistance to ground of 100 of these insulators? (b) Calculate the power dissipated by 100 of them. (c) What fraction of the power carried by the line is this? Explicitly show how you follow the steps in the Problem-Solving Strategies for Series and Parallel Resistors .

The diagram shows a grounded metal transmission tower. Two ground conductors on top of the tower point out like antennas. Hanging from the tower are a set of three bundled conductors, one on either end and one in the middle.
High-voltage (240-kV) transmission line carrying 5.00 × 10 2 A is hung from a grounded metal transmission tower. The row of ceramic insulators provide 1.00 × 10 9 Ω size 12{1 "." 0 times "10" rSup { size 8{9} } %OMEGA } {} of resistance each.

Show that if two resistors R 1 size 12{R rSub { size 8{1} } } {} and R 2 size 12{R rSub { size 8{2} } } {} are combined and one is much greater than the other ( R 1 >> R 2 size 12{R rSub { size 8{1} } ">>"R rSub { size 8{2} } } {} ): (a) Their series resistance is very nearly equal to the greater resistance R 1 size 12{R rSub { size 8{1} } } {} . (b) Their parallel resistance is very nearly equal to smaller resistance R 2 size 12{R rSub { size 8{2} } } {} .

(a) R s = R 1 + R 2 ⇒ R s ≈ R 1 R 1 >> R 2 alignl { stack { size 12{R rSub { size 8{s} } =R rSub { size 8{1} } +R rSub { size 8{2} } } {} #drarrow R rSub { size 8{s} } »R rSub { size 8{1} } left (R rSub { size 8{1} } ">>"R rSub { size 8{2} } right ) {} } } {}

(b) 1 R p = 1 R 1 + 1 R 2 = R 1 + R 2 R 1 R 2 size 12{ { {1} over {R rSub { size 8{p} } } } = { {1} over {R rSub { size 8{1} } } } + { {1} over {R rSub { size 8{2} } } } = { {R rSub { size 8{1} } +R rSub { size 8{2} } } over {R rSub { size 8{1} } R rSub { size 8{2} } } } } {} ,

so that

R p = R 1 R 2 R 1 + R 2 ≈ R 1 R 2 R 1 = R 2 R 1 >> R 2 . alignl { stack { size 12{R rSub { size 8{p} } = { {R rSub { size 8{1} } R rSub { size 8{2} } } over {R rSub { size 8{1} } +R rSub { size 8{2} } } } » { {R rSub { size 8{1} } R rSub { size 8{2} } } over {R rSub { size 8{1} } } } } {} #=R rSub { size 8{2} } left (R rSub { size 8{1} } ">>"R rSub { size 8{2} } right ) "." {} } } {}

Unreasonable Results

Two resistors, one having a resistance of 1 45 Ω size 12{1"45 " %OMEGA } {} , are connected in parallel to produce a total resistance of 150 Ω size 12{150 %OMEGA } {} . (a) What is the value of the second resistance? (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?

Unreasonable Results

Two resistors, one having a resistance of 9 00 kΩ size 12{9"00 k" %OMEGA } {} , are connected in series to produce a total resistance of 0 . 500 MΩ size 12{0 "." "500 M" %OMEGA } {} . (a) What is the value of the second resistance? (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?

(a) - 400 k Ω size 12{ +- "400 k" %OMEGA } {}

(b) Resistance cannot be negative.

(c) Series resistance is said to be less than one of the resistors, but it must be greater than any of the resistors.
<< Chapter < Page Page > Chapter >>
Practice Key Terms 9

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

Get it on Google Play Download on the App Store Now




Source:  OpenStax, Physics 101. OpenStax CNX. Jan 07, 2013 Download for free at http://legacy.cnx.org/content/col11479/1.1
Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Physics 101' conversation and receive update notifications?

Ask