| << Chapter < Page | Chapter >> Page > |
The power of a lens is defined to be the inverse of its focal length. In equation form, this is
where is the focal length of the lens, which must be given in meters (and not cm or mm). The power of a lens has the unit diopters (D), provided that the focal length is given in meters. That is, , or . (Note that this power (optical power, actually) is not the same as power in watts. It is a concept related to the effect of optical devices on light.) Optometrists prescribe common spectacles and contact lenses in units of diopters.
Suppose you take a magnifying glass out on a sunny day and you find that it concentrates sunlight to a small spot 8.00 cm away from the lens. What are the focal length and power of the lens?
Strategy
The situation here is the same as those shown in [link] and [link] . The Sun is so far away that the Sun’s rays are nearly parallel when they reach Earth. The magnifying glass is a convex (or converging) lens, focusing the nearly parallel rays of sunlight. Thus the focal length of the lens is the distance from the lens to the spot, and its power is the inverse of this distance (in m).
Solution
The focal length of the lens is the distance from the center of the lens to the spot, given to be 8.00 cm. Thus,
To find the power of the lens, we must first convert the focal length to meters; then, we substitute this value into the equation for power. This gives
Discussion
This is a relatively powerful lens. The power of a lens in diopters should not be confused with the familiar concept of power in watts. It is an unfortunate fact that the word “power” is used for two completely different concepts. If you examine a prescription for eyeglasses, you will note lens powers given in diopters. If you examine the label on a motor, you will note energy consumption rate given as a power in watts.
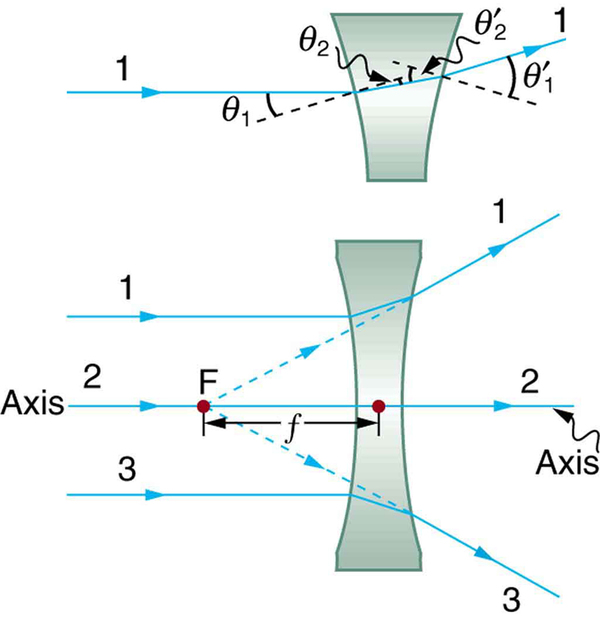
[link] shows a concave lens and the effect it has on rays of light that enter it parallel to its axis (the path taken by ray 2 in the figure is the axis of the lens). The concave lens is a diverging lens , because it causes the light rays to bend away (diverge) from its axis. In this case, the lens has been shaped so that all light rays entering it parallel to its axis appear to originate from the same point, , defined to be the focal point of a diverging lens. The distance from the center of the lens to the focal point is again called the focal length of the lens. Note that the focal length and power of a diverging lens are defined to be negative. For example, if the distance to in [link] is 5.00 cm, then the focal length is and the power of the lens is . An expanded view of the path of one ray through the lens is shown in the figure to illustrate how the shape of the lens, together with the law of refraction, causes the ray to follow its particular path and be diverged.

Notification Switch
Would you like to follow the 'Concepts of physics with linear momentum' conversation and receive update notifications?