This module is from Fundamentals of Mathematics by Denny Burzynski and Wade Ellis, Jr. This module discusses how to add and subtract fractions with like denominators. By the end of the module students should be able to add and subtract fractions with like denominators.
Section overview
- Addition of Fraction With Like Denominators
- Subtraction of Fractions With Like Denominators
Addition of fraction with like denominators
Let's examine the following diagram.

2 one-fifths and 1 one fifth is shaded.
It is shown in the shaded regions of the diagram that
(2 one-fifths) + (1 one-fifth) = (3 one-fifths)
That is,
From this observation, we can suggest the following rule.
Method of adding fractions having like denominators
To add two or more fractions that have the same denominators, add the numerators and place the resulting sum over the common denominator. Reduce, if necessary.
Sample set a
Find the following sums.
. The denominators are the same. Add the numerators and place that sum over 7.
. The denominators are the same. Add the numerators and place the sum over 8. Reduce.
. The denominators are the same. Add the numerators and place the sum over 9.
. The denominators are the same. Add the numerators and place the sum over 8.
To see what happens if we
mistakenly add the denominators as well as the numerators, let's add
Adding the numerators and
mistakenly adding the denominators produces
This means that two
's is the same as one
. Preposterous!
We do not add denominators .
Practice set a
Find the following sums.
Show why adding both the numerators and denominators is preposterous by adding
and
and examining the result.
, so two
’s= one
which is preposterous.
Subtraction of fractions with like denominators
We can picture the concept of subtraction of fractions in much the same way we pictured addition.
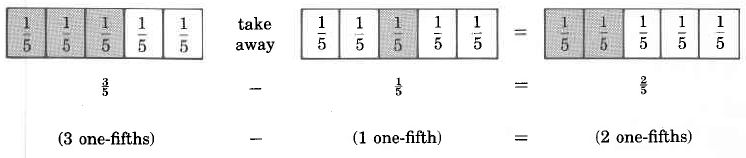
From this observation, we can suggest the following rule for subtracting fractions having like denominators:
Subtraction of fractions with like denominators
To subtract two fractions that have like denominators, subtract the numerators and place the resulting difference over the common denominator. Reduce, if possible.
Sample set b
Find the following differences.
. The denominators are the same. Subtract the numerators. Place the difference over 5.
. The denominators are the same. Subtract the numerators. Place the difference over 6.
. The denominators are the same. Subtract numerators and place the difference over 9.
To see what happens if we
mistakenly subtract the denominators, let's consider
We get division by zero, which is undefined.
We do not subtract denominators.
Practice set b
Find the following differences.
Show why subtracting both the numerators and the denominators is in error by performing the subtraction
.
, which is undefined
Exercises
For the following problems, find the sums and differences. Be sure to reduce.
The following rule for addition and subtraction of two fractions is preposterous. Show why by performing the operations using the rule for the following two problems.
Preposterous rule
To add or subtract two fractions, simply add or subtract the numerators and place this result over the sum or difference of the denominators.
(using the preposterous rule)
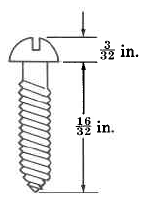
Find the total length of the screw.
Two months ago, a woman paid off
of a loan. One month ago, she paid off
of the total loan. This month she will again pay off
of the total loan. At the end of the month, how much of her total loan will she have paid off?
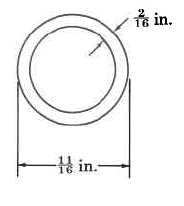
Find the inside diameter of the pipe.
Exercises for review
(
[link] ) Round 2,650 to the nearest hundred.
(
[link] ) Use the numbers 2, 4, and 8 to illustrate the associative property of addition.
(
[link] ) Find the prime factors of 495.
(
[link] ) Find the value of
.
(
[link] )
of what number is
?




