| << Chapter < Page | Chapter >> Page > |
Now, let’s rearrange Newton’s second law to solve for acceleration. We get
In this form, it’s clear that acceleration is directly proportional to force, which we write as
where the symbol SYMBOL means “proportional to.”
This proportionality states mathematically what we just said in words—acceleration is directly proportional to the net external force. When two variables are directly proportional to each other, then if one variable doubles, the other variable must double. Likewise, if one variable is reduced by half, the other variable must also be reduced by half. In general, when one variable is multiplied by a number, the other variable will also be multiplied by the same number. It seems reasonable that the acceleration of a system should be directly proportional to and in the same direction as the net external force acting on the system. An object experiences greater acceleration when acted on by a greater force.
It is also clear from the equation that acceleration is inversely proportional to mass, which we write as
Inversely proportional means that, if one variable is multiplied by a number, the other variable must be divided by the same number. Now, it also seems reasonable that acceleration should be inversely proportional to the mass of the system. In other words, the larger the mass (the inertia), the smaller the acceleration produced by a given force. This is illustrated in Figure 04_03_basketball, which shows that a given net external force applied to a basketball produces a much greater acceleration than when applied to a car.
| Forces | Equations |
|---|---|
| Newton's second law of motion | |
| acceleration |
|
| mass |
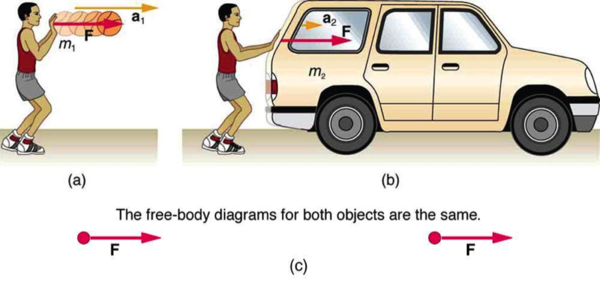
The same force exerted on systems of different mass produces different accelerations. (a) A boy pushes a basketball to make a pass. The effect of gravity on the ball is ignored. (b) The same boy pushing with identical force on a stalled car produces a far smaller acceleration (friction is negligible). Note that the free-body diagrams for the ball and for the car are identical, which allows us to compare the two situations.
[BL] Review how to convert between units.
[OL]
[AL] Ask students to give examples of Newton’s Second Law.
[BL] [OL] [AL] Talk about people’s weight. Ask them what they think they would weigh on the moon. Talk about the difference between mass and weight.
Before putting Newton’s second law into action, it is important to consider units. The equation F net = ma is used to define the units of force in terms of the three basic units of mass, length, and time (recall that acceleration has units of length divided by time squared). The SI unit of force is called the newton (abbreviated N) and is the force needed to accelerate a 1 kg system at the rate of . That is, because , we have

Notification Switch
Would you like to follow the 'Updated tutor hs physics content - legacy' conversation and receive update notifications?