| << Chapter < Page | Chapter >> Page > |
Displacement is a measurement of change in position of the particle in motion. Its magnitude and direction are measured by the length and direction of the straight line joining initial and final positions of the particle. Obviously, the length of the straight line between the positions is the shortest distance between the points.
From physical view point, displacement conveys the meaning of shortest distance plus direction of the motion between two time instants or corresponding two positions. Initial and final positions of the point object are the only important consideration for measuring magnitude of displacement. Actual path between two positions has no consequence in so far as displacement is concerned.
The quantum of displacement is measured by the length of the straight line joining two ends of motion. If there is no change in the position at the end of a motion, the displacement is zero.
In order to illustrate the underlying concept of displacement, let us consider the motion of a particle from A to B to C. The displacement vector is represented by the vector AC and its magnitude by the length of AC.
Displacement
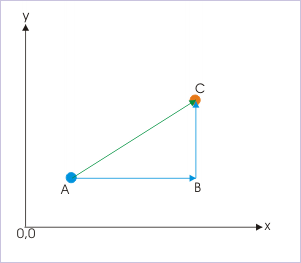
Once motion has begun, magnitude of displacement may increase or decrease (at a slow, fast or constant rate) or may even be zero, if the object returns to its initial position. Since a body under motion can take any arbitrary path, it is always possible that the end point of the motion may come closer or may go farther away from the initial point. Thus, displacement, unlike distance, may decrease from a given level.
In order to understand the variations in displacement with the progress of motion, let us consider another example of the motion of a particle along the rectangular path from A to B to C to D to A. Magnitude of displacement, shown by dotted vectors, is increasing during motion from A to B to C. Whereas magnitude of displacement is decreasing as the particle moves from C to D to A, eventually being equal to zero, when the particle returns to A.
Displacement

However, displacement is essentially a measurement of length combined with direction. As direction has no dimension, its dimensional formula is also [L] like that of distance; and likewise, its SI measurement unit is ‘meter’.
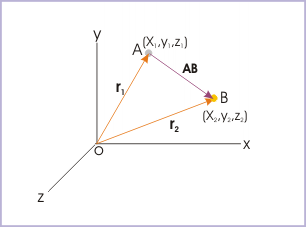
We have the liberty to describe displacement vector as an independent vector ( AB ) or in terms of position vectors ( and ). The choice depends on the problem in hand. The description, however, is equivalent.
Let us consider that a point object moves from point A (represented by position vector ) to point B (represented by position vector ) as shown in the figure. Now, using triangle law (moving from O to A to B to O), we have :
Displacement in terms of position vectors

Notification Switch
Would you like to follow the 'Physics for k-12' conversation and receive update notifications?