0.6 Equilibrium and the second law of thermodynamics  (Page 2/11)

We conclude that, although there is no energetic advantage to the dye molecules dispersing themselves,they do so spontaneously. Furthermore, this process is irreversible in the sense that, without considerable effort on our part, the dye molecules will never return to form asingle localized drop. We now seek an understanding of how and whythis mixing occurs.

Consider the following rather abstract model for the dye molecules in the water. For the glass, we take a row often small boxes, each one of which represents a possible location for a molecule, either of water or of dye. For the molecules, wetake marbles, clear for water and blue for ink. Each box will accommodate only a single marble, since two molecules cannot be inthe same place as the same time. Since we see a drop of dye when the molecules are congregated, we model a "drop" as three bluemarbles in consecutive boxes. Notice that there are only eight ways to have a "drop" of dye, assuming that the three dye "molecules"are indistinguishable from one another. Two possibilities are shown in [link] and [link] . It is not difficult to find the other six.

Arrangement of three ink molecules

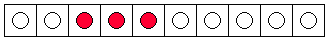
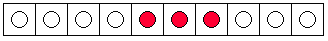
By contrast, there are many more ways to arrange the dye molecules so that they do not form a drop, i.e. , so that the three molecules are not together. Two possibilities are shown in [link] and [link] . The total number of such possibilities is 112. (The total number of all possiblearrangements can be calculated as follows: there are 10 possible locations for the first blue marble, 9 for the second, and 8 forthe third. This gives 720 possible arrangements, but many of these are identical, since the marbles are indistinguishable. The numberof duplicates for each arrangement is 6, calculated from three choices for the first marble, two for the second, and one for thethird. The total number of non-identical arrangements of the molecules is $\frac{720}{6}=120$ .) We conclude that, if we randomly place the 3 marbles in the tray of10 boxes, the chances are only 8 out of 120 (or 1 out of 15) of observing a drop of ink.

Now, in a real experiment, there are many, many times more ink molecules and many, many times more possiblepositions for each molecule. To see how this comes into play, consider a row of 500 boxes and 5 blue marbles. (The mole fraction of ink is thus 0.01.) The total number of distinct configurations of the blue marbles in these boxes isapproximately $2E11$ . The number of these configurations which have all five ink marblestogether in a drop is 496. If the arrangements are sampled randomly, the chances of observing a drop of ink with all fivemolecules together are thus about one in 500 million. The possibilities are remote even for observing a partial "droplet"consisting of fewer than all five dye molecules. The chance for four of the molecules to be found together is about one in 800,000.Even if we define a droplet to be only three molecules together, the chances of observing one are less than one in 1600.

We could, with some difficulty, calculate the probability for observing a drop of ink when there are $10^{23}$ molecules. However, it is reasonably deduced from our small calculations that the probability is essentially zero for the inkmolecules, randomly distributed into the water molecules, to be found together. We conclude from this that the reason why weobserve ink to disperse in water is that the probability is infinitesimally small for randomly distributed dye molecules to becongregated in a drop.