0.15 Planetary motion

The trajectory of motion resulting from general solution of “two body” system is a conic section. Subject to initial velocities and relative mass, eccentricity of conic section can have different values. We interpret a conic section for different eccentricity to represent different types of trajectories. We have already discussed straight line and circular trajectories. In this module, we shall discuss elliptical trajectory, which is the trajectory of a planet in the solar system.

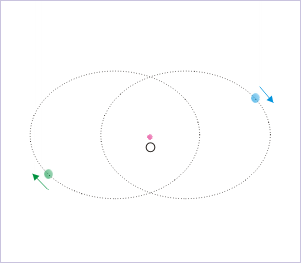
In a general scenario of “two body system”, involving elliptical trajectory, each body revolves around the common “center of mass” called “barycenter”. The two elliptical paths intersect, but bodies are not at the point of intersection at the same time and as such there is no collision.

Two body system

In this module, however, we shall keep our focus on the planetary motion and Kepler’s planetary laws. We are basically seeking to describe planetary motion – particularly that in our solar system. The trajectory of planet is elliptical with one qualification. The Sun, being many times heavier than the planets, is almost at rest in the reference frame of motion. It lies at one of the foci of the elliptical path of the planets around it. Here, we assume that center of mass is about same as the center of Sun. Clearly, planetary motion is a special case of elliptical motion of “two body system” interacted by mutual attraction.

We should, however, be aware that general solution of planetary motion involves second order differential equation, which is solved using polar coordinates.

Ellipse

We need to learn about the basics of elliptical trajectory and terminology associated with it. It is important from the point of view of applying laws of Newtonian mechanics. We shall, however, be limited to the basics only.

Conic section

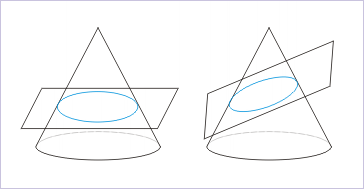
Conic section is obtained by the intersection of a plane with a cone. Two such intersections, one for a circle and one for an ellipse are shown in the figure.

Conic sections

Elliptical trajectory

Here, we recount the elementary geometry of an ellipse in order to understand planetary motion. The equation of an ellipse centered at the origin of a rectangular coordinate (0,0) is :

$$\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$$

where “a” is semi-major axis and “b” is semi-minor axis as shown in the figure.

Ellipse

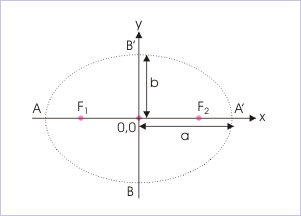
Note that “ $F_1$ ” and “ $F_2$ ” are two foci of the ellipse.

Eccentricity

The eccentricity of a conic section is measure of “how different it is from a circle”. Higher the eccentricity, greater is deviation. The eccentricity (e) of a conic section is defined in terms of “a” and “b” as :

$$e=\sqrt{\left(1-\frac{k{b}^2}{a^2}\right)}$$

where “k” is 1 for an ellipse, 0 for parabola and -1 for hyperbola. The values of eccentricity for different trajectories are as give here :

1. The eccentricity of a straight line is 1, if we consider b=0 for the straight line.
2. The eccentricity of an ellipse falls between 0 and 1.
3. The eccentricity of a circle is 0
4. The eccentricity of a parabola is 1.
5. The eccentricity of a hyperbola is greater than 1.