0.1 Rational expression concepts -- simplifying rational expressions

How do you simplify a fraction? The answer is, you divide the top and bottom by the same thing.

So $\frac{4}{6}$ and $\frac{2}{3}$ are two different ways of writing the same number.

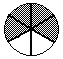On the left, a pizza divided into six equal slices: the four shaded-in regions represent $\frac{4}{6}$ of a pizza. On the right, a pizza divided into three equal slices: the two shaded-in regions represent $\frac{2}{3}$ of a pizza. The two areas are identical: $\frac{4}{6}$ and $\frac{2}{3}$ are two different ways of expressing the same amount of pizza .

In some cases, you have to repeat this process more than once before the fraction is fully simplified.

It is vital to remember that we have not divided this fraction by 4, or by 2, or by 8 . We have rewritten the fraction in another form: $\frac{\text{40}}{\text{48}}$ is the same number as $\frac{5}{6}$ . In strictly practical terms, if you are given the choice between $\frac{\text{40}}{\text{48}}$ of a pizza or $\frac{5}{6}$ of a pizza, it does not matter which one you choose, because they are the same amount of pizza.

You can divide the top and bottom of a fraction by the same number, but you cannot subtract the same number from the top and bottom of a fraction!

$\frac{\text{40}}{\text{48}}=\frac{\text{40}-\text{39}}{\text{48}-\text{39}}=\frac{1}{9}$ $✗$ Wrong!

Given the choice, a hungry person would be wise to choose $\frac{\text{40}}{\text{48}}$ of a pizza instead of $\frac{1}{9}$ .

Dividing the top and bottom of a fraction by the same number leaves the fraction unchanged, and that is how you simplify fractions. Subtracting the same number from the top and bottom changes the value of the fraction, and is therefore an illegal simplification.

All this is review. But if you understand these basic fraction concepts, you are ahead of many Algebra II students! And if you can apply these same concepts when variables are involved , then you are ready to simplify rational expressions, because there are no new concepts involved.

As an example, consider the following:

You might at first be tempted to cancel the common $x^2$ terms on the top and bottom. But this would be, mathematically, subtracting $x^2$ from both the top and the bottom; which, as we have seen, is an illegal fraction operation.

$\frac{x^2-9}{x^2+\mathrm{6x}+9}$

$=\frac{-9}{\mathrm{6x}+9}$

$✗$ Wrong!

To properly simplify this expression, begin by factoring both the top and the bottom, and then see if anything cancels.

What we have created, of course, is an algebraic generalization:

For any x value, the complicated expression on the left will give the same answer as the much simpler expression on the right. You may want to try one or two values, just to confirm that it works.

As you can see, the skills of factoring and simplifying fractions come together in this exercise. No new skills are required.