| << Chapter < Page | Chapter >> Page > |

You start by multiplying by to get
Then you multiply by lining up the partial product in the correct columns.
Last, you add the partial products.
Now we'll apply this same method to multiply two binomials.
Multiply using the vertical method:
It does not matter which binomial goes on the top. Line up the columns when you multiply as we did when we multiplied
|
| |
| Multiply by . |
|
| Multiply by . |
|
| Add like terms. |
|
Notice the partial products are the same as the terms in the FOIL method.
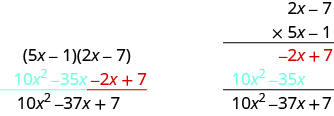
We have now used three methods for multiplying binomials. Be sure to practice each method, and try to decide which one you prefer. The three methods are listed here to help you remember them.
We have multiplied monomials by monomials, monomials by polynomials, and binomials by binomials. Now we're ready to multiply a trinomial by a binomial. Remember, the FOIL method will not work in this case, but we can use either the Distributive Property or the Vertical Method . We first look at an example using the Distributive Property.
Multiply using the Distributive Property:
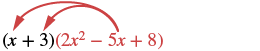 | |
| Distribute. |
|
| Multiply. | |
| Combine like terms. |
Multiply using the Distributive Property:
y 3 − 8 y 2 + 9 y − 2
Multiply using the Distributive Property:
3 x 3 + 2 x 2 − 3 x + 10
Now let's do this same multiplication using the Vertical Method.
Multiply using the Vertical Method:
It is easier to put the polynomial with fewer terms on the bottom because we get fewer partial products this way.
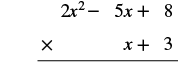 | |
| Multiply by 3. |
|
| Multiply by . |
|
| Add like terms. |
|
Multiply using the Vertical Method:
3 x 3 + 2 x 2 − 3 x + 10
| Step 1. Multiply the First terms. |
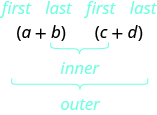 |
| Step 2. Multiply the Outer terms. | |
| Step 3. Multiply the Inner terms. | |
| Step 4. Multiply the Last terms. | |
| Step 5. Combine like terms, when possible. |
Multiply a Polynomial by a Monomial
In the following exercises, multiply.
Multiply a Binomial by a Binomial
In the following exercises, multiply the following binomials using different methods.
Multiply a Trinomial by a Binomial
In the following exercises, using either method.
Mental math You can use binomial multiplication to multiply numbers without a calculator. Say you need to multiply times Think of as and as
Mental math You can use binomial multiplication to multiply numbers without a calculator. Say you need to multiply times Think of as and as
Which method do you prefer to use when multiplying two binomials—the Distributive Property, the FOIL method, or the Vertical Method? Why?
Answers will vary.
Which method do you prefer to use when multiplying a trinomial by a binomial—the Distributive Property or the Vertical Method? Why?
ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
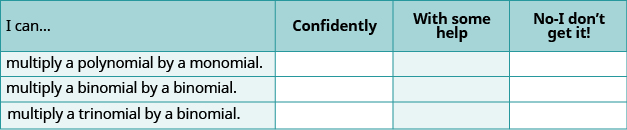
ⓑ What does this checklist tell you about your mastery of this section? What steps will you take to improve?

Notification Switch
Would you like to follow the 'Prealgebra' conversation and receive update notifications?