| << Chapter < Page | Chapter >> Page > |
Binary division follow the same rules as in decimal division.
Logical Operation with one or two bits
NOT : Changes the value of a single bit. If it is a "1", the result is "0"; if it is a "0", the result is "1".
AND: Compares 2 bits and if they are both "1", then the result is "1", otherwise, the result is "0".
OR : Compares 2 bits and if either or both bits are "1", then the result is "1", otherwise, the result is "0".
XOR : Compares 2 bits and if exactly one of them is "1" (i.e., if they are different values), then the result is "1"; otherwise (if the bits are the same), the result is "0".
Logical operators between two bits have the following truth table
| x | y | x AND y | x OR y | x XOR y |
| 1 | 1 | 1 | 1 | 0 |
| 1 | 0 | 0 | 1 | 1 |
| 0 | 1 | 0 | 1 | 1 |
| 0 | 0 | 0 | 0 | 0 |
Logical Operation with one or two binary numbers
A logical (bitwise) operation operates on one or two bit patterns or binary numerals at the level of their individual bits.
Example
NOT 0111
= 1000
AND operation
An AND operation takes two binary representations of equal length and performs the logical AND operation on each pair of corresponding bits. In each pair, the result is 1 if the first bit is 1 AND the second bit is 1. Otherwise, the result is 0.
Example
0101
AND 0011= 0001
OR operation
An OR operation takes two bit patterns of equal length, and produces another one of the same length by matching up corresponding bits (the first of each; the second of each; and so on) and performing the logical OR operation on each pair of corresponding bits.
Example
0101
OR 0011= 0111
XOR Operation
An exclusive or operation takes two bit patterns of equal length and performs the logical XOR operation on each pair of corresponding bits.
Example
0101XOR 0011
= 0110
It is important to handle character data. Character data is not just alphabetic characters, but also numeric characters, punctuation, spaces, etc. They need to be represented in binary.
There aren't mathematical properties for character data, so assigning binary codes for characters is somewhat arbitrary.
ASCII Code Table
ASCII stands for American Standard Code for Information Interchange. The ASCII standard was developed in 1963, permitted machines from different manufacturers to exchange data.
ASCII code table consists of 128 binary values (0 to 127), each associated with a character or command. The non-printing characters are used to control peripherals such as printer.
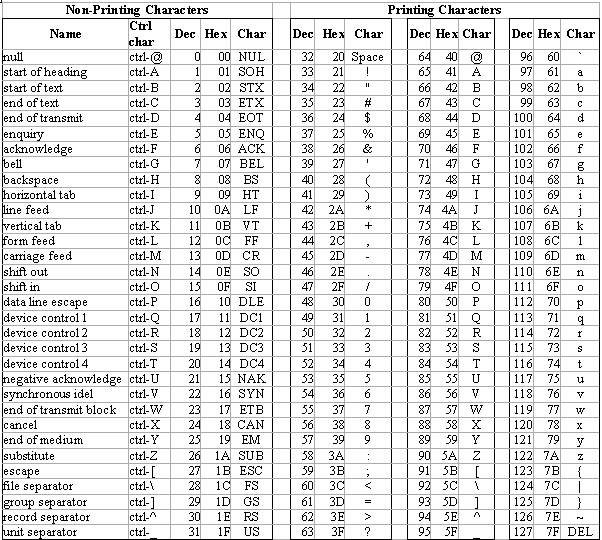
The extended ASCII character set also consists 128 128 characters representing additional special, mathematical, graphic and foreign characters.

There are some problems with the ASCII code table. With ASCII character set, string datatypes allocated one byte per character. But logographic languages such as Chinese, Japanese, and Korean need far more than 256 characters for reasonable representation. Even Vietnamese, a language uses almost Latin letters, need 61 characters for representation. Where can we find numbers for our characters? is it a solution : 2 bytes per character?

Notification Switch
Would you like to follow the 'Introduction to computer science' conversation and receive update notifications?