2.4 Accelerated motion in two dimensions  (Page 5/6)

Application

Questions and their answers are presented here in the module text format as if it were an extension of the treatment of the topic. The idea is to provide a verbose explanation, detailing the application of theory. Solution presented is, therefore, treated as the part of the understanding process – not merely a Q/A session. The emphasis is to enforce ideas and concepts, which can not be completely absorbed unless they are put to real time situation.

Representative problems and their solutions

We discuss problems, which highlight certain aspects of the study leading to the accelerated motion in two dimensions. The questions are categorized in terms of the characterizing features of the subject matter :

• Path of motion
• Tangential and normal accelerations
• Nature of motion
• Displacement in two dimensions

Path of motion

Problem : A balloon starts rising from the surface with a constant upward velocity, “ $v_0$ ”. The balloon gains a horizontal drift due to the wind. The horizontal drift velocity is given by “ky”, where “k” is a constant and “y” is the vertical height of the balloon from the surface. Derive an expression of path of the motion.

Solution : An inspection of the equation of drift velocity (v = ky) suggests that balloon drifts more with the gain in height. A suggestive x-y plot of the motion is shown here.

Motion of a balloon

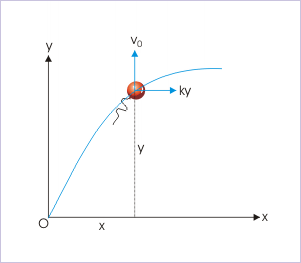
Let vertical and horizontal direction corresponds to “y” and “x” axes of the coordinate system. Here,

$$v_y=v_0$$

$$v_x=k_y$$

We are required to know the relation between vertical and horizontal components of displacement from the expression of component velocities. It means that we need to know a lower order attribute from higher order attribute. Thus, we shall proceed with integration of differential equation, which defines velocity as :

$$\Rightarrow \frac{đx}{đt}=ky$$

Similarly,

$$\frac{đy}{đt}=v_0$$

$$\Rightarrow đy=v_0đt$$

Combining two equations by eliminating “dt”,

$$dx=\frac{kyđy}{v_0}$$

Now, integrating both sides, we have :

$$x=\int \frac{kyđy}{v_0}$$

Taking out constants out of the integral,

$$\Rightarrow x=\frac{k}{v_0}\int yđy$$

$$x=\frac{k{y}^2}{2v_0}$$

This is the required equation of motion, which is an equation of a parabola. Thus, the suggested plot given in the beginning, as a matter of fact, was correct.

Got questions? Get instant answers now!

Tangential and normal accelerations

Problem : A balloon starts rising from the surface with a constant upward velocity, “ $v_0$ ”. The balloon gains a horizontal drift due to the wind. The horizontal drift velocity is given by “ky”, where “k” is a constant and “y” is the vertical height of the balloon from the surface. Derive expressions for the tangential and normal accelerations of the balloon.

Solution : We can proceed to find the magnitude of total acceleration by first finding the expression of velocity. Here, velocity is given as :

$$v=kyi+v_{0}j$$

Since acceleration is higher order attribute, we obtain its expression by differentiating the expression of velocity with respect to time :

$$\Rightarrow a=\frac{đv}{đt}=k{v}_{y}i=k{v}_{0}i$$

It is obvious that acceleration is one dimensional. It is evident from the data given also. The balloon moving with constant vertical velocity has no acceleration in y-direction. The speed of the balloon in x-direction, however, keeps changing with height (time) and as such total acceleration of the balloon is in x-direction. The magnitude of total acceleration is :