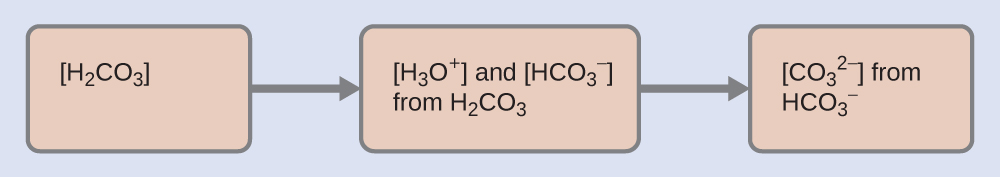14.5 Polyprotic acids  (Page 2/8)

Solution

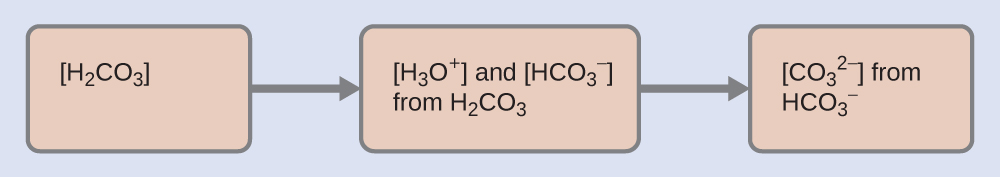
1. Determine the concentrations of $H_{\mathit{3}}{O}^{\mathit{\text{+}}}$ and $HC{O}_{\mathit{3}}{}^{\mathit{\text{−}}}.$
   
   ${\text{H}}_2{\text{CO}}_3(aq)+{\text{H}}_2\text{O}(l)\rightleftharpoons {\text{H}}_3{\text{O}}^{\text{+}}(aq)+{\text{HCO}}_3{}^{\text{−}}(aq)K_{\text{a}1}=4.3\times {10}^{\mathrm{-7}}$
   
   As for the ionization of any other weak acid:
   
   An abbreviated table of changes and concentrations shows:
   
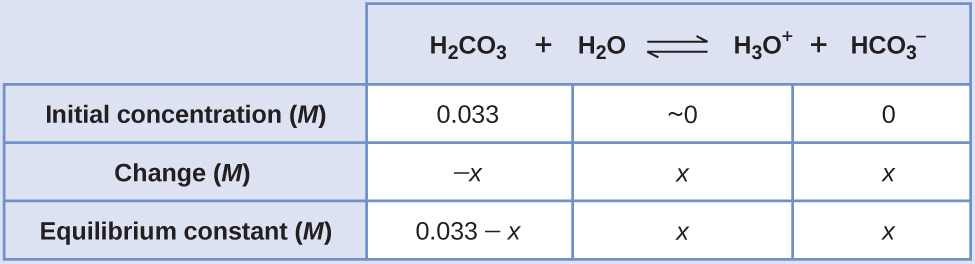
   Substituting the equilibrium concentrations into the equilibrium gives us:
   
   $K_{{\text{H}}_2{\text{CO}}_3}=\frac{[{\text{H}}_3{\text{O}}^{\text{+}}][{\text{HCO}}_3{}^{\text{−}}]}{[{\text{H}}_2{\text{CO}}_3]}=\frac{(x)(x)}{0.033-x}=4.3\times {10}^{\mathrm{-7}}$
   
   Solving the preceding equation making our standard assumptions gives:
   
   $x=1.2\times {10}^{\mathrm{-4}}$
   
   Thus:
   
   $[{\text{H}}_2{\text{CO}}_3]=0.033M$
   
   
   $[{\text{H}}_3{\text{O}}^{\text{+}}]=[{\text{HCO}}_3{}^{\text{−}}]=1.2\times {10}^{\mathrm{-4}}M$
2. Determine the concentration of $C{O}_{\mathit{3}}{}^{\mathrm{2-}}$ in a solution at equilibrium with $\mathit{[}{H}_{\mathit{3}}{O}^{\mathit{\text{+}}}\mathit{]}$ and $\mathit{[}HC{O}_{\mathit{3}}{}^{\mathit{\text{−}}}\mathit{]}$ both equal to 1.2 $\times$ 10 ⁻⁴ M .
   
   ${\text{HCO}}_3{}^{\text{−}}(aq)+{\text{H}}_2\text{O}(l)\rightleftharpoons {\text{H}}_3{\text{O}}^{\text{+}}(aq)+{\text{CO}}_3{}^{\mathrm{2-}}(aq)$
   
   
   $K_{{\text{HCO}}_3{}^{\text{−}}}=\frac{[{\text{H}}_3{\text{O}}^{\text{+}}][{\text{CO}}_3{}^{\mathrm{2-}}]}{[{\text{HCO}}_3{}^{\text{−}}]}=\frac{(1.2\times {10}^{\mathrm{-4}})[{\text{CO}}_3{}^{\mathrm{2-}}]}{1.2\times {10}^{\mathrm{-4}}}$
   
   
   $[{\text{CO}}_3{}^{\mathrm{2-}}]=\frac{(5.6\times {10}^{\mathrm{-11}})(1.2\times {10}^{\mathrm{-4}})}{1.2\times {10}^{\mathrm{-4}}}=5.6\times {10}^{\mathrm{-11}}M$

To summarize: In part 1 of this example, we found that the H ₂ CO ₃ in a 0.033- M solution ionizes slightly and at equilibrium [H ₂ CO ₃ ] = 0.033 M ; $[{\text{H}}_3{\text{O}}^{\text{+}}]$ = 1.2 $\times$ 10 ⁻⁴ ; and $[{\text{HCO}}_3{}^{\text{−}}]=1.2\times {10}^{\mathrm{-4}}M.$ In part 2, we determined that $[{\text{CO}}_3{}^{\mathrm{2-}}]=5.6\times {10}^{\mathrm{-11}}M.$

Check your learning

A triprotic acid    is an acid that has three dissociable protons that undergo stepwise ionization: Phosphoric acid is a typical example:

As with the diprotic acids, the differences in the ionization constants of these reactions tell us that in each successive step the degree of ionization is significantly weaker. This is a general characteristic of polyprotic acids and successive ionization constants often differ by a factor of about 10 ⁵ to 10 ⁶ .

This set of three dissociation reactions may appear to make calculations of equilibrium concentrations in a solution of H ₃ PO ₄ complicated. However, because the successive ionization constants differ by a factor of 10 ⁵ to 10 ⁶ , the calculations can be broken down into a series of parts similar to those for diprotic acids.

Polyprotic bases can accept more than one hydrogen ion in solution. The carbonate ion is an example of a diprotic base    , since it can accept up to two protons. Solutions of alkali metal carbonates are quite alkaline, due to the reactions: