| << Chapter < Page | Chapter >> Page > |
Find a linear equation to solve for the following unknown quantities: One number is three more than twice another number. If the sum of the two numbers is find the numbers.
11 and 25
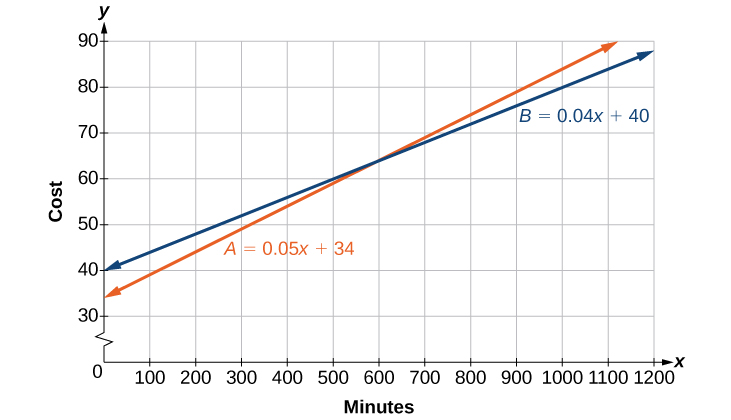
There are two cell phone companies that offer different packages. Company A charges a monthly service fee of $34 plus $.05/min talk-time. Company B charges a monthly service fee of $40 plus $.04/min talk-time.
If the average number of minutes used each month is 1,160, we have the following:
So, Company B offers the lower monthly cost of $86.40 as compared with the $92 monthly cost offered by Company A when the average number of minutes used each month is 1,160.
If the average number of minutes used each month is 420, we have the following:
If the average number of minutes used each month is 420, then Company A offers a lower monthly cost of $55 compared to Company B ’s monthly cost of $56.80.
To answer the question of how many talk-time minutes would yield the same bill from both companies, we should think about the problem in terms of coordinates: At what point are both the x- value and the y- value equal? We can find this point by setting the equations equal to each other and solving for x.
Check the x- value in each equation.
Therefore, a monthly average of 600 talk-time minutes renders the plans equal. See [link]
Find a linear equation to model this real-world application: It costs ABC electronics company $2.50 per unit to produce a part used in a popular brand of desktop computers. The company has monthly operating expenses of $350 for utilities and $3,300 for salaries. What are the company’s monthly expenses?
Many applications are solved using known formulas. The problem is stated, a formula is identified, the known quantities are substituted into the formula, the equation is solved for the unknown, and the problem’s question is answered. Typically, these problems involve two equations representing two trips, two investments, two areas, and so on. Examples of formulas include the area of a rectangular region, the perimeter of a rectangle, and the volume of a rectangular solid, When there are two unknowns, we find a way to write one in terms of the other because we can solve for only one variable at a time.

Notification Switch
Would you like to follow the 'College algebra' conversation and receive update notifications?