6.7 Exponential and logarithmic models  (Page 2/16)

A General Note

Characteristics of the exponential function, y = A ₀ e ^kt

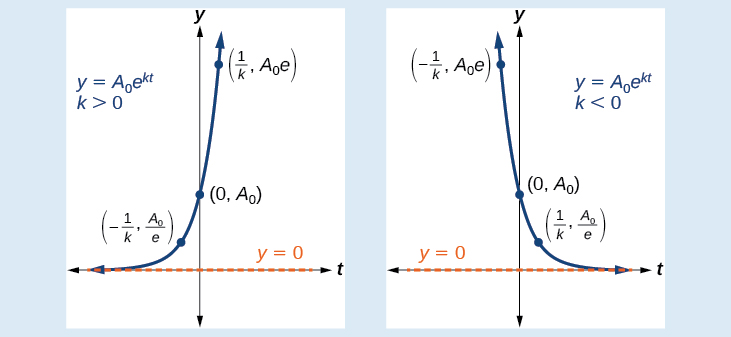
An exponential function with the form $y=A_0{e}^{kt}$ has the following characteristics:

• one-to-one function
• horizontal asymptote: $y=0$
• domain: $(–\infty , \infty )$
• range: $(0,\infty )$
• x intercept: none
• y-intercept: $\left(0,A_0\right)$
• increasing if $k>0$ (see [link] )
• decreasing if $k<0$ (see [link] )

Graphing exponential growth

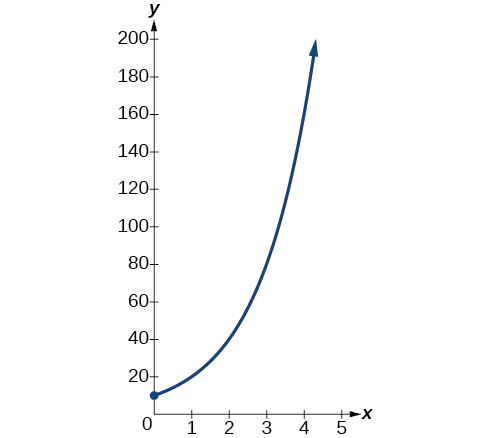
A population of bacteria doubles every hour. If the culture started with 10 bacteria, graph the population as a function of time.

When an amount grows at a fixed percent per unit time, the growth is exponential. To find $A_0$ we use the fact that $A_0$ is the amount at time zero, so $A_0=10.$ To find $k,$ use the fact that after one hour $\left(t=1\right)$ the population doubles from $10$ to $20.$ The formula is derived as follows

$$\begin{array}{ll}20=10e^{k\cdot 1}\hfill & \hfill \\ 2=e^k\hfill & \text{Divide by 10}\hfill \\ \ln 2=k\hfill & \text{Take the natural logarithm}\hfill \end{array}$$

so $k=\ln (2).$ Thus the equation we want to graph is $y=10e^{(\ln 2)t}=10{(e^{\ln 2})}^t=10·2^t.$ The graph is shown in [link] .

Got questions? Get instant answers now!

Got questions? Get instant answers now!

Half-life

We now turn to exponential decay . One of the common terms associated with exponential decay, as stated above, is half-life , the length of time it takes an exponentially decaying quantity to decrease to half its original amount. Every radioactive isotope has a half-life, and the process describing the exponential decay of an isotope is called radioactive decay.

To find the half-life of a function describing exponential decay, solve the following equation:

We find that the half-life depends only on the constant $k$ and not on the starting quantity $A_0.$

The formula is derived as follows

Since $t,$ the time, is positive, $k$ must, as expected, be negative. This gives us the half-life formula

Radiocarbon dating

The formula for radioactive decay is important in radiocarbon dating , which is used to calculate the approximate date a plant or animal died. Radiocarbon dating was discovered in 1949 by Willard Libby, who won a Nobel Prize for his discovery. It compares the difference between the ratio of two isotopes of carbon in an organic artifact or fossil to the ratio of those two isotopes in the air. It is believed to be accurate to within about 1% error for plants or animals that died within the last 60,000 years.