| << Chapter < Page | Chapter >> Page > |
Show that the function has at least two real zeros between and
As a start, evaluate at the integer values and See [link] .
| 1 | 2 | 3 | 4 | |
| 5 | 0 | –3 | 2 |
We see that one zero occurs at Also, since is negative and is positive, by the Intermediate Value Theorem, there must be at least one real zero between 3 and 4.
We have shown that there are at least two real zeros between and
Show that the function has at least one real zero between and
Because is a polynomial function and since is negative and is positive, there is at least one real zero between and
Now that we know how to find zeros of polynomial functions, we can use them to write formulas based on graphs. Because a polynomial function written in factored form will have an x -intercept where each factor is equal to zero, we can form a function that will pass through a set of x -intercepts by introducing a corresponding set of factors.
If a polynomial of lowest degree has horizontal intercepts at then the polynomial can be written in the factored form: where the powers on each factor can be determined by the behavior of the graph at the corresponding intercept, and the stretch factor can be determined given a value of the function other than the x -intercept.
Given a graph of a polynomial function, write a formula for the function.
Write a formula for the polynomial function shown in [link] .
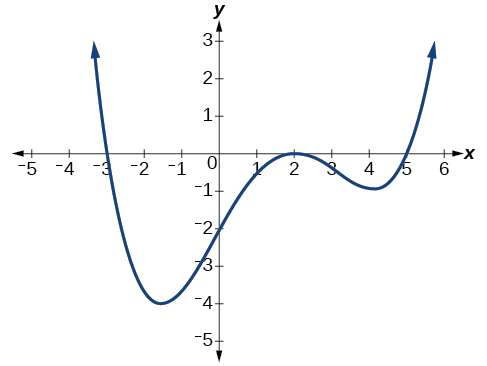
This graph has three x -intercepts: and The y -intercept is located at At and the graph passes through the axis linearly, suggesting the corresponding factors of the polynomial will be linear. At the graph bounces at the intercept, suggesting the corresponding factor of the polynomial will be second degree (quadratic). Together, this gives us
To determine the stretch factor, we utilize another point on the graph. We will use the intercept to solve for
The graphed polynomial appears to represent the function
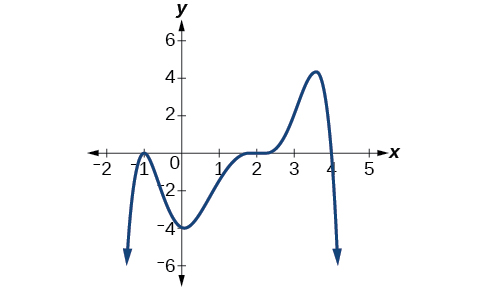
Given the graph shown in [link] , write a formula for the function shown.
With quadratics, we were able to algebraically find the maximum or minimum value of the function by finding the vertex. For general polynomials, finding these turning points is not possible without more advanced techniques from calculus. Even then, finding where extrema occur can still be algebraically challenging. For now, we will estimate the locations of turning points using technology to generate a graph.

Notification Switch
Would you like to follow the 'Algebra and trigonometry' conversation and receive update notifications?